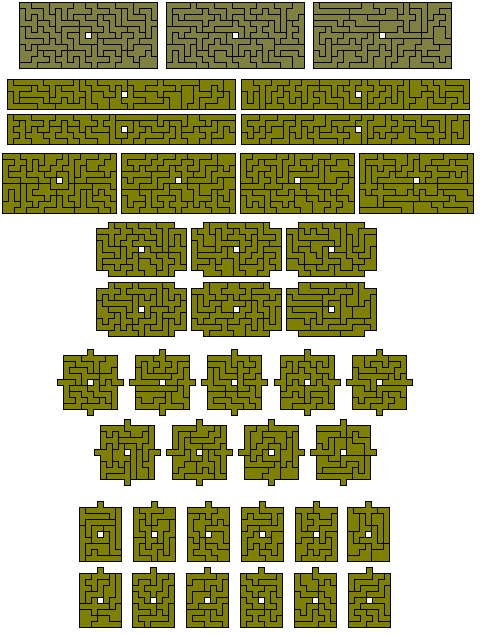
Patrick Hamlyn has produced the following solution for twelve 8x8 squares.
Heptomino Constructions
A number of sets of congruent shapes is possible with 3, 4, 6 and 9 parts. For rectangles with a single hole the possibilities are - three 11x23; four 5x38 or 10x19; nine 5x17; twelve 4x16 or 8x8.

Patrick Hamlyn has produced the following solution for twelve 8x8 squares.

If we omit the piece with the hole then we get 107 heptominoes which, since 107 is prime, can form only a 7x107 rectangle.
A number of multiple replications of pieces is also possible with this set.
If we consider the one-sided heptominoes then we have 196 pieces with one piece with a hole.
Omitting the piece with the hole could allow a number of rectangles to be formed. The 35x39 is shown here as well as three 7x65 and three 13x35 rectangles which provide solutions to the 21x65, 7x195 and 13x105rectangles and finally a 15x91 rectangle.
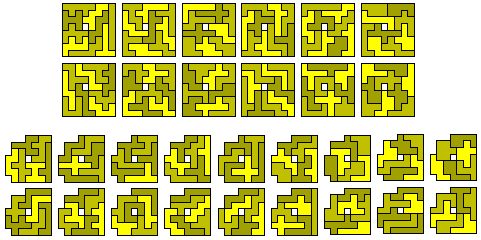
Patrick Hamlyn has found fifteen 7x13 rectangles made with this set shown here in three coloured format. Patrick has also found three 5x91 rectangles giving solutions for 5x273 and 15x91 rectangles. The only other rectangle with the correct area is the 3x455 which is not possible. He has also found nineteen 7x10 and one 7x5 rectangles.