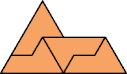

Reptiles are known for polyominoes, polyiamonds, polyaboloes, and other polyforms.
Few polyforms of any kind form reptiles. A bireptile is a figure of which copies can be joined to form two joined, equally enlarged copies of the original figure.
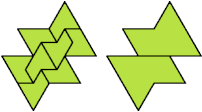
Any figure with a reptiling trivially has a bireptiling, but not every figure with a bireptiling has a reptiling. That is, bireptiles are more common than reptiles.
Below I show minimal known bireptilings for various polyiamonds.
| Number of Cells | Number of Reptiles | Number
of Bireptiles |
|---|---|---|
| 1 | 1 | 1 |
| 2 | 1 | 1 |
| 3 | 1 | 1 |
| 4 | 2 | 2 |
| 5 | 1 | 3 |
| 6 | 4 | 5 |
| 7 | 0 | 1 |
| 8 | 7 | 8 |
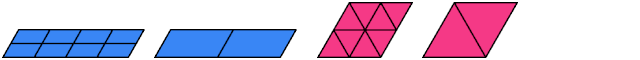

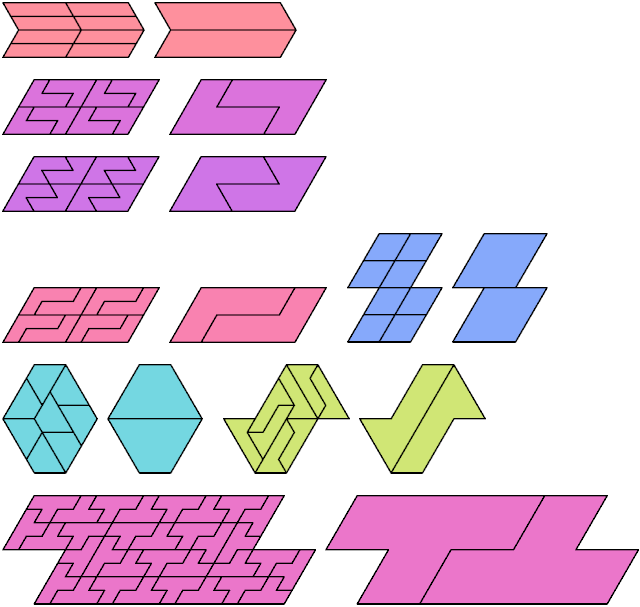
Yoshiaki Araki has found that the J pentiamond can form a bireptile at any scale that is a multiple of 5. Click here to see his first few cases.
Click here for a bireptile at scale 4.
Click here for ten bireptiles at scale 5.
The J pentiamond also has bireptilings at scales 6, 7, and 8.


Last revised 2022-12-07.