Tetracube-Pentacube Pair Oddities

Introduction

A tetracube is a solid made of 4 equal cubes joined face to face. There are 8 tetracubes, counting distinct mirror images.

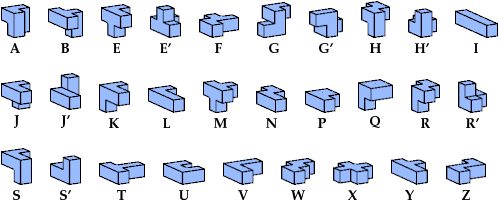
A pentacube is a solid made of 5 equal cubes joined face to face. There are 29 pentacubes, counting distinct mirror images.

A polyform oddity or Sillke Figure is a polyform with binary symmetry at least, tiled by an odd number of copies of a given polyform.

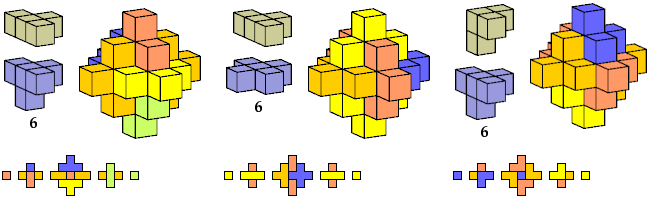
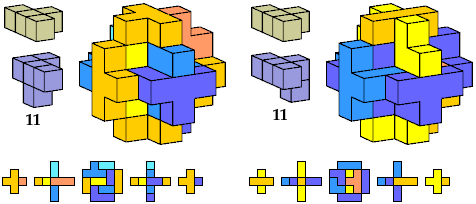
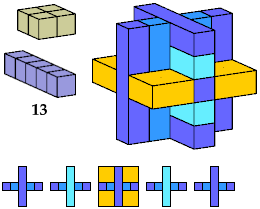
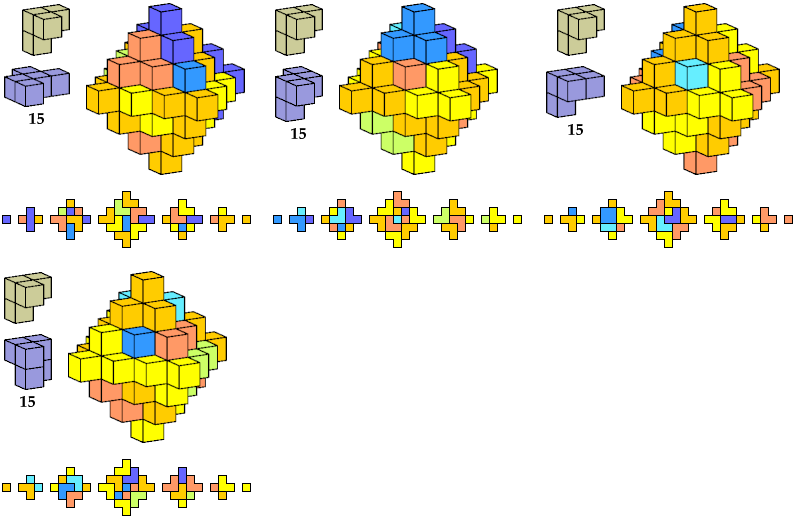
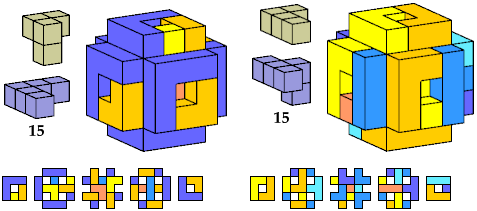
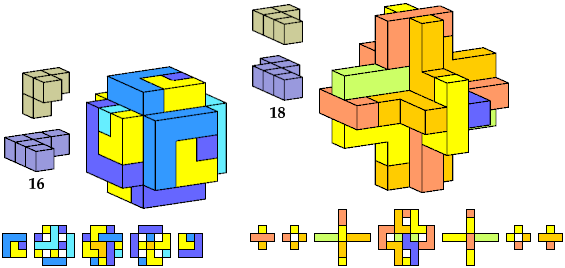
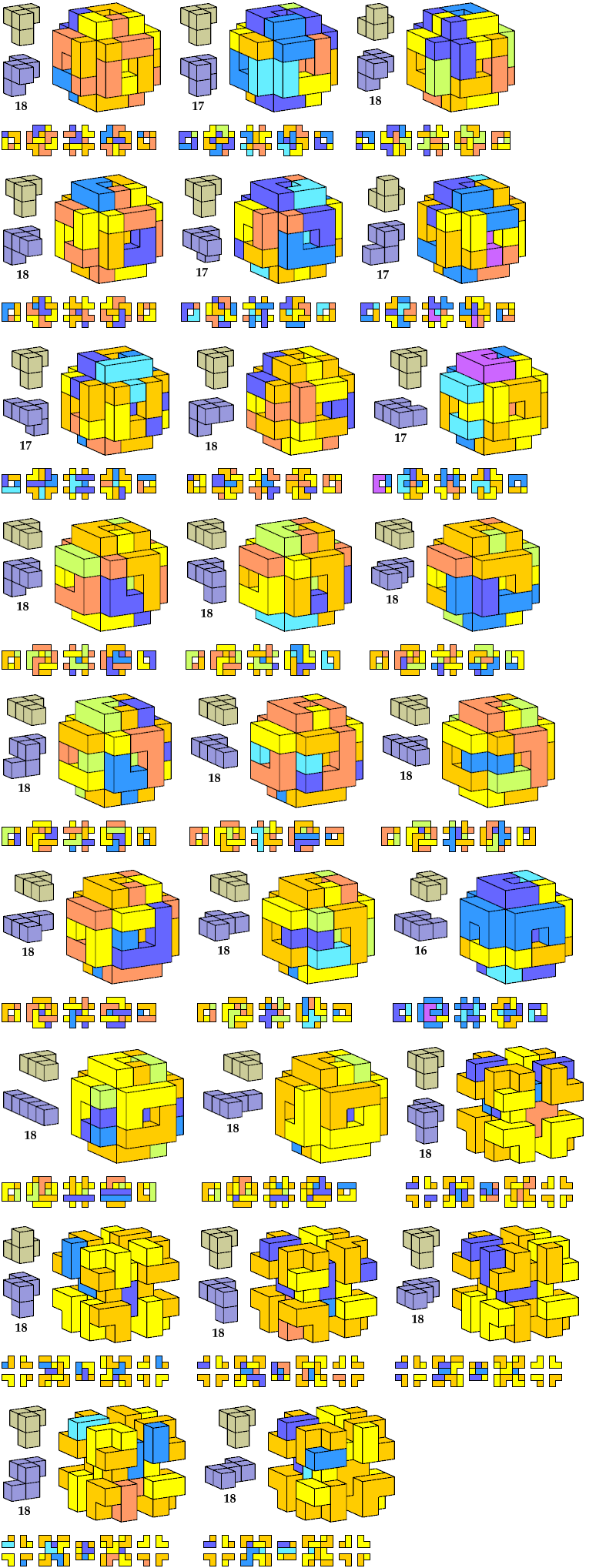
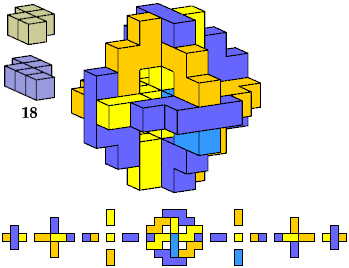
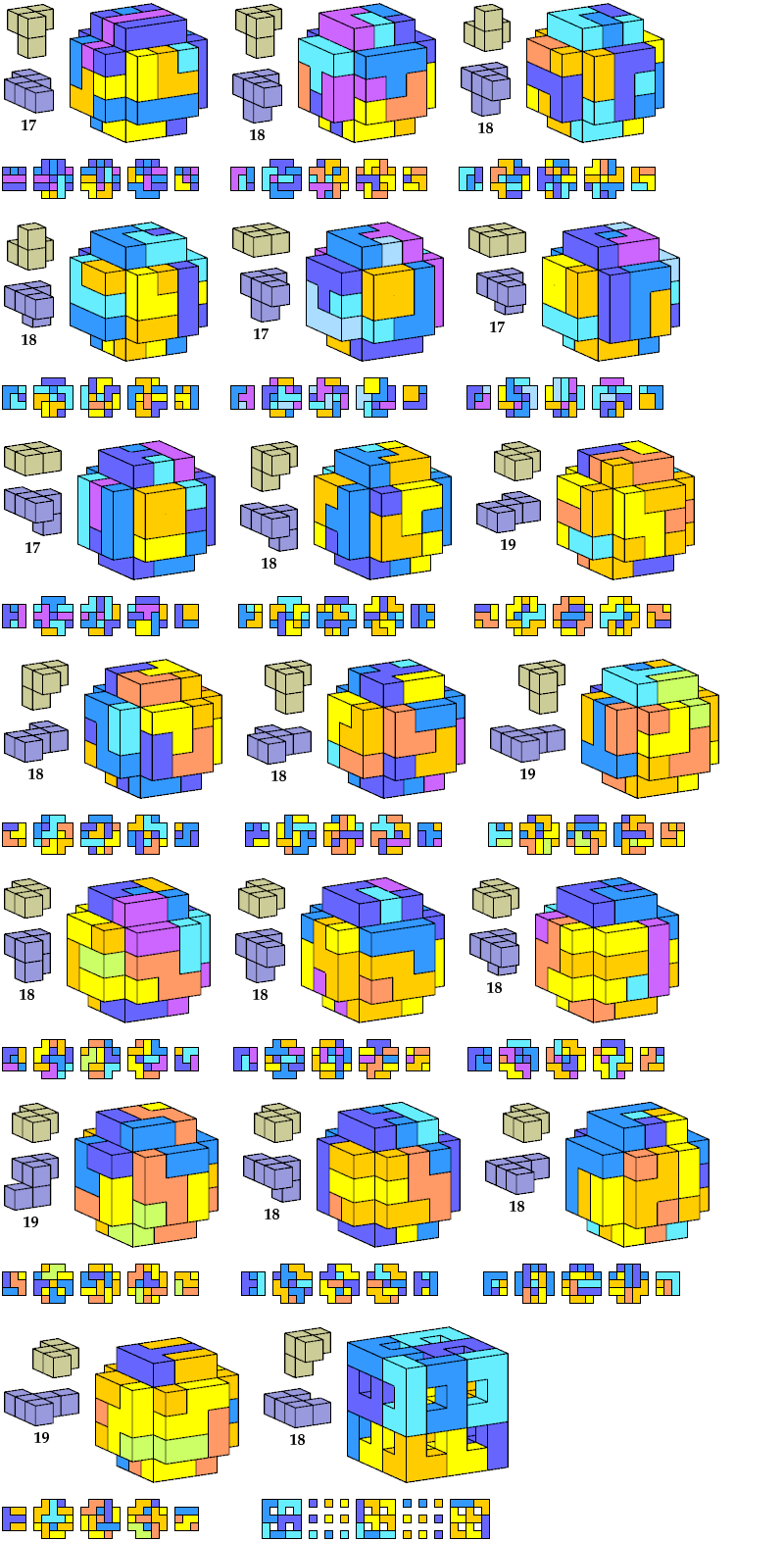
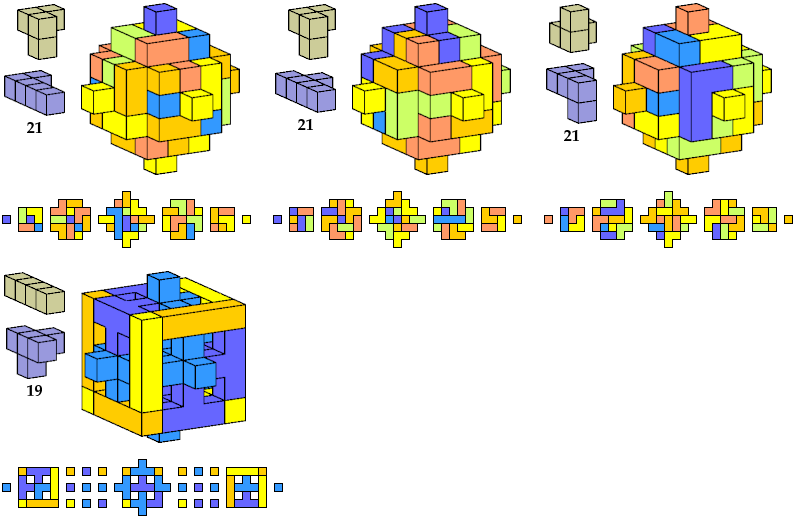
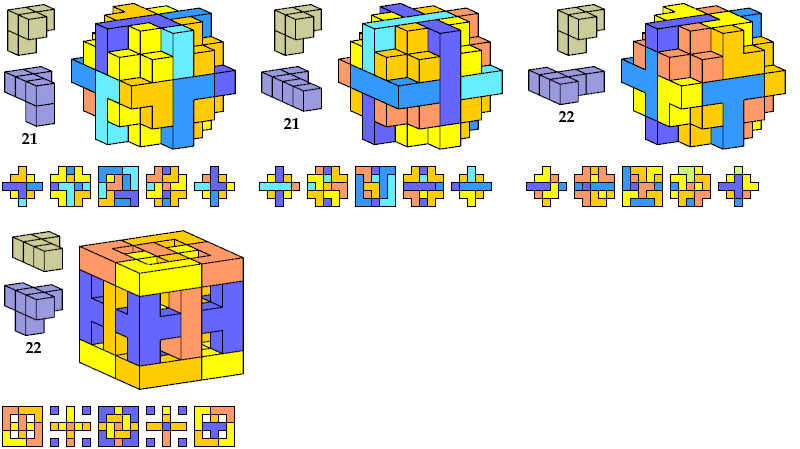
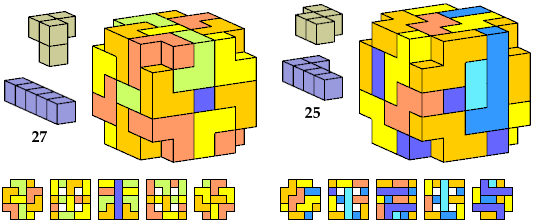
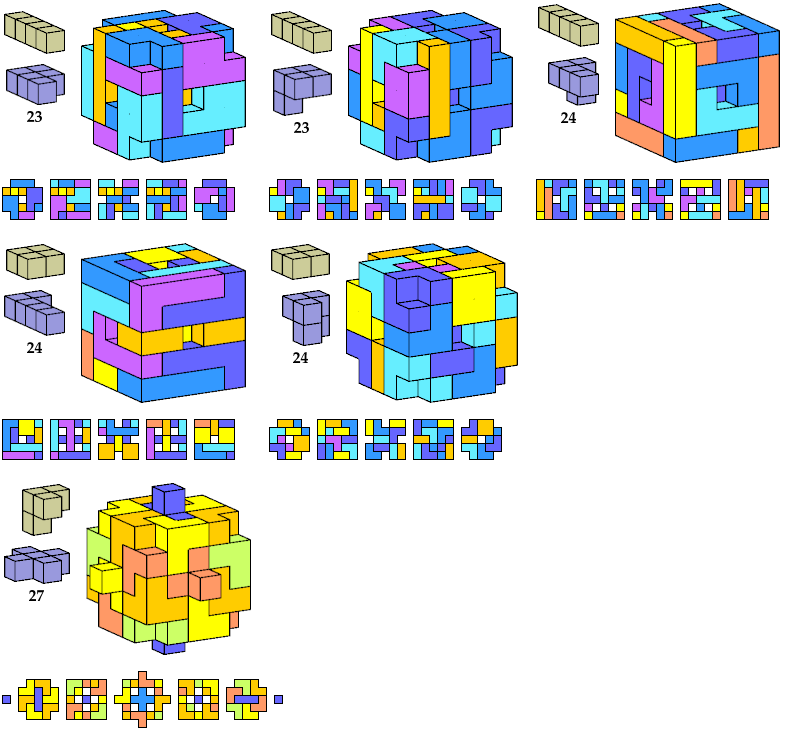
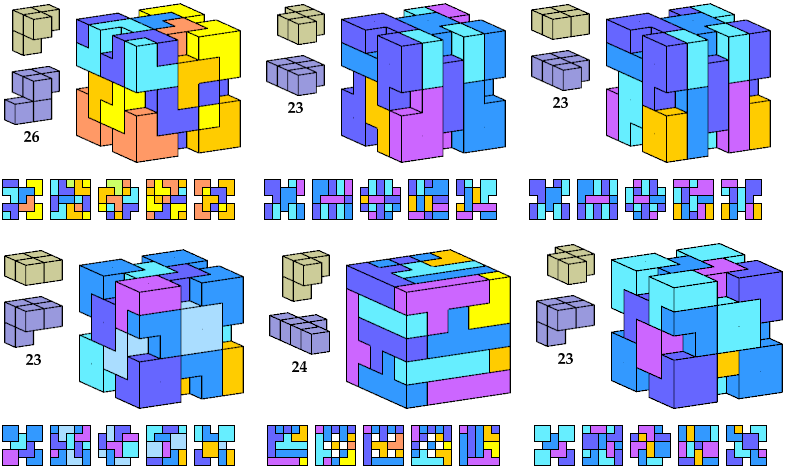
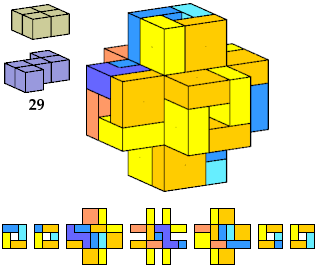
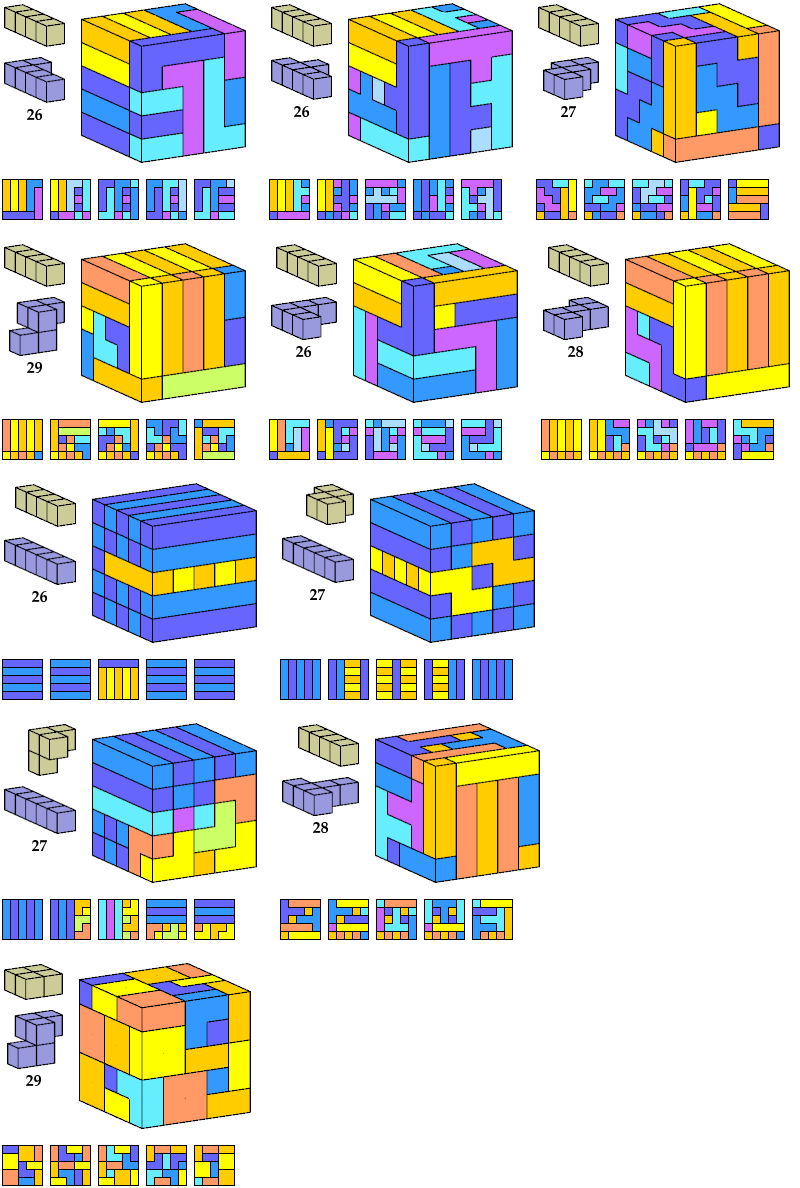
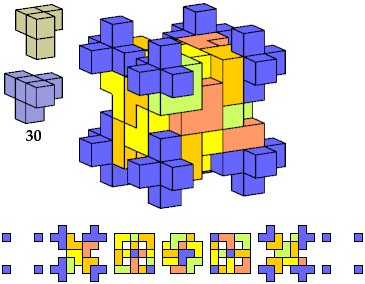
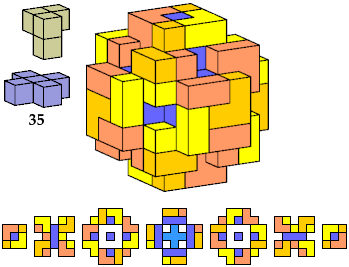
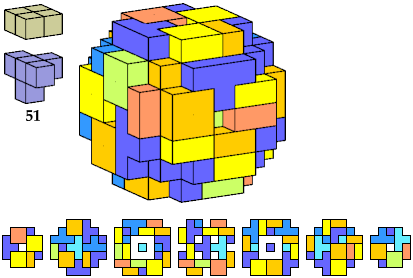
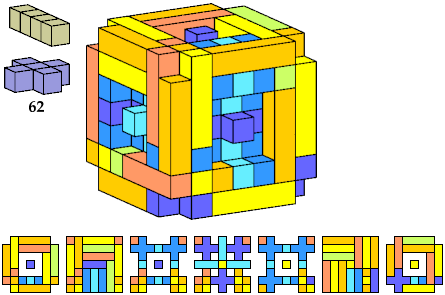
Here I show polycubes with full symmetry and an odd number of cells, formed by joining copies of a given tetracube and a given pentacube. The solutions are the smallest known to me. The figures shown are the numbers of tiles used.

If you find a smaller solution, please write.

Table of Results

	A	B	E	F	G	H	I	J	K	L	M	N	P	Q	R	S	T	U	V	W	X	Y	Z
I	119	119	119	117	125	117	125	111	117	125	87	117	111	111	117	117	125	117	125	125	267	125	125
K	63	81	27	63	113	57	125	27	57	93	25	57	27	63	63	93	93	81	73	27	111	113	81
L	27	67	27	75	75	27	75	27	75	75	93	73	27	27	27	75	75	27	75	75	105	75	27
N	81	81	81	99	81	117	125	81	119	109	117	79	113	113	117	117	81	75	81	117	117	105	81
Q	111	81	81	117	125	117	61	81	119	111	225	117	113	113	117	117	117	119	27	213	?	117	123
S	75	75	81	81	75	75	109	75	75	87	129	81	27	75	75	75	81	75	67	75	147	87	75
S′	75	75	81	81	75	75	109	81	75	87	129	81	27	75	75	87	81	75	67	75	147	87	75
T	57	49	49	57	57	57	57	57	57	57	25	57	27	57	57	57	57	27	57	57	25	57	57