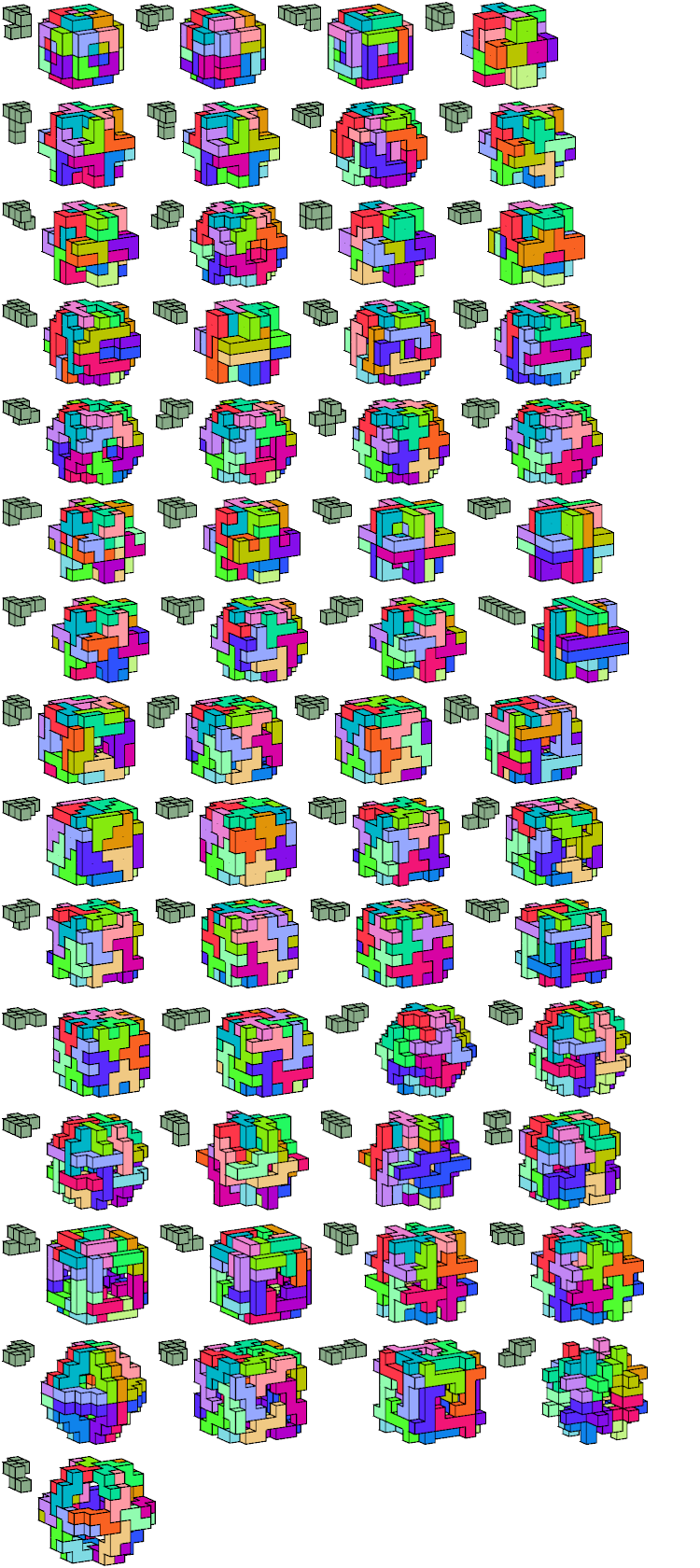
Baiocchi Figures for Polycubes
A Baiocchi figure is a figure
formed by joining copies of a polyform and having the maximal
symmetry for the polyform's class.
For polycubes, that means cubic symmetry.
Claudio
Baiocchi proposed the idea in January 2008.
Baiocchi figures first appeared in Erich Friedman's
Math Magic for that month.
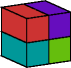
Below are minimal known Baiocchi figures for polycubes
of orders 1 through 5.
Not all these solutions are uniquely minimal.
For Baiocchi figures for pentacubes with an odd number of tiles,
see Pentacube Oddities.

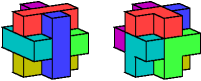
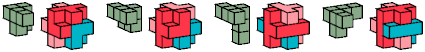
 The solutions for four chiral hexacubes marked with an asterisk
(*) use reflected tiles.
See below for variant solutions that
do not require reflection.
The solutions for four chiral hexacubes marked with an asterisk
(*) use reflected tiles.
See below for variant solutions that
do not require reflection.
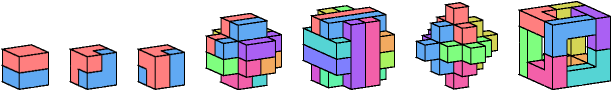
3 Tiles
8 Tiles
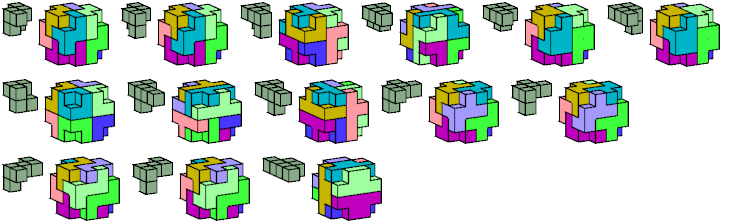
12 Tiles

16 Tiles
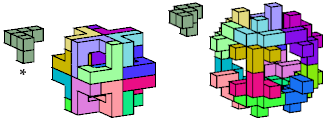
18 Tiles
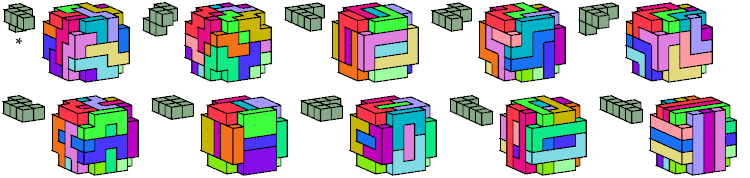
20 Tiles
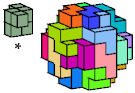
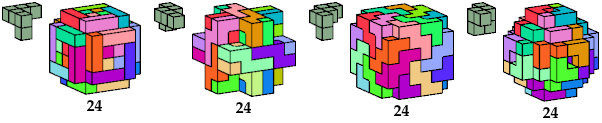
24 Tiles
Last revised 2016-01-22.
Back to Polyform Compatibility
<
Baiocchi Figures
<
Polyform Curiosities
Col. George Sicherman
[ HOME
| MAIL
]












