
On this page, for each pair of pentahexes, I show a frame tiling with the fewest known cells, using at least one copy of each pentahex of the pair. If you find a smaller solution or solve an unsolved case, please write.
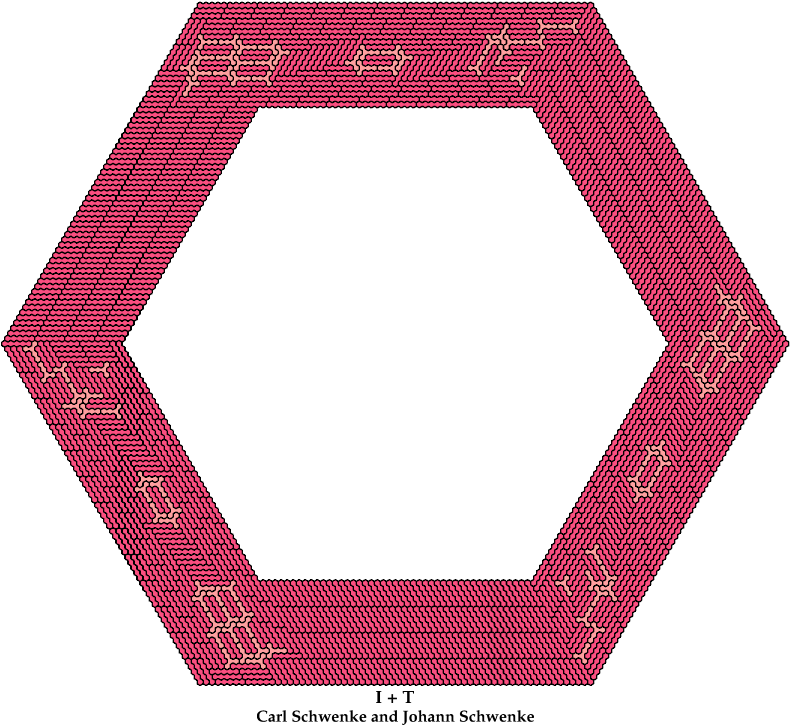
Carl Schwenke and Johann Schwenke solved the pairs 5I+5X and 5I+5T.

| A | C | D | E | F | H | I | J | K | L | N | P | Q | R | S | T | U | V | W | X | Y | Z | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A | • | 12 | 12 | 12 | 18 | 24 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 126 | 90 | 24 | 24 | 114 | 12 | 12 | 18 |
| C | 12 | • | 12 | 54 | 12 | 12 | 12 | 90 | 12 | 6 | 12 | 12 | 18 | 18 | — | — | — | 24 | 6 | 12 | 12 | 12 |
| D | 12 | 12 | • | 6 | 12 | 12 | 12 | 12 | 12 | 6 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 18 | 12 | 12 | 12 |
| E | 12 | 54 | 6 | • | 12 | 12 | 18 | 12 | 12 | 12 | 12 | 12 | 18 | 12 | 108 | 144 | 24 | 6 | 18 | 12 | 18 | 18 |
| F | 18 | 12 | 12 | 12 | • | 18 | 12 | 66 | 18 | 12 | 18 | 12 | 12 | 12 | — | — | — | 42 | 66 | 12 | 12 | 18 |
| H | 24 | 12 | 12 | 12 | 18 | • | 24 | 12 | — | 12 | 12 | 12 | — | 18 | — | — | 12 | 66 | — | — | 12 | — |
| I | 12 | 12 | 12 | 18 | 12 | 24 | • | 30 | 24 | 12 | 12 | 12 | 24 | 18 | 42 | 1332 | 30 | 12 | 54 | 522 | 12 | 18 |
| J | 12 | 90 | 12 | 12 | 66 | 12 | 30 | • | 12 | 12 | 12 | 12 | 18 | 12 | — | 36 | — | 12 | — | 12 | 6 | 12 |
| K | 12 | 12 | 12 | 12 | 18 | — | 24 | 12 | • | 6 | 12 | 12 | — | 18 | — | — | 24 | 12 | — | — | 12 | — |
| L | 12 | 6 | 6 | 12 | 12 | 12 | 12 | 12 | 6 | • | 12 | 6 | 6 | 6 | 18 | 18 | 12 | 12 | 12 | 12 | 6 | 6 |
| N | 12 | 12 | 12 | 12 | 18 | 12 | 12 | 12 | 12 | 12 | • | 6 | 12 | 12 | 12 | 12 | 18 | 12 | 18 | 12 | 12 | 12 |
| P | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 6 | 6 | • | 6 | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 6 | 12 |
| Q | 12 | 18 | 12 | 18 | 12 | — | 24 | 18 | — | 6 | 12 | 6 | • | 30 | — | — | 18 | 18 | — | — | 12 | — |
| R | 12 | 18 | 12 | 12 | 12 | 18 | 18 | 12 | 18 | 6 | 12 | 12 | 30 | • | — | — | 18 | 24 | 18 | 78 | 12 | 12 |
| S | 126 | — | 12 | 108 | — | — | 42 | — | — | 18 | 12 | 12 | — | — | • | — | — | 6 | — | — | 12 | — |
| T | 90 | — | 12 | 144 | — | — | 1332 | 36 | — | 18 | 12 | 12 | — | — | — | • | 12 | 6 | — | — | 18 | — |
| U | 24 | — | 12 | 24 | — | 12 | 30 | — | 24 | 12 | 18 | 12 | 18 | 18 | — | 12 | • | 42 | — | 18 | 12 | 24 |
| V | 24 | 24 | 12 | 6 | 42 | 66 | 12 | 12 | 12 | 12 | 12 | 12 | 18 | 24 | 6 | 6 | 42 | • | 42 | 72 | 12 | 12 |
| W | 114 | 6 | 18 | 18 | 66 | — | 54 | — | — | 12 | 18 | 12 | — | 18 | — | — | — | 42 | • | — | 12 | — |
| X | 12 | 12 | 12 | 12 | 12 | — | 522 | 12 | — | 12 | 12 | 12 | — | 78 | — | — | 18 | 72 | — | • | 12 | — |
| Y | 12 | 12 | 12 | 18 | 12 | 12 | 12 | 6 | 12 | 6 | 12 | 6 | 12 | 12 | 12 | 18 | 12 | 12 | 12 | 12 | • | 12 |
| Z | 18 | 12 | 12 | 18 | 18 | — | 18 | 12 | — | 6 | 12 | 12 | — | 12 | — | — | 24 | 12 | — | — | 12 | • |

















Last revised 2025-09-10.