Heptiamond Compatibility

Introduction

A heptiamond is a figure made of seven equilateral triangles joined edge to edge. There are 24 such figures, not distinguishing reflections and rotations.

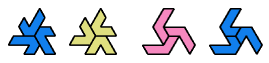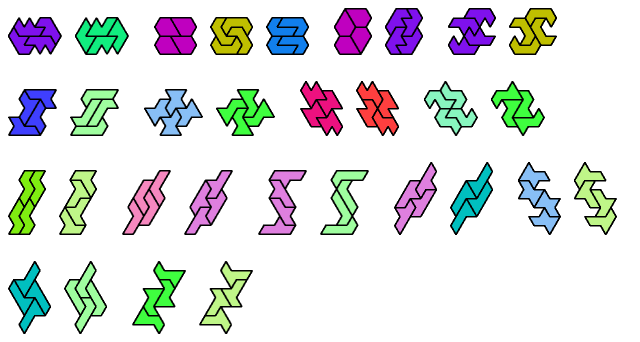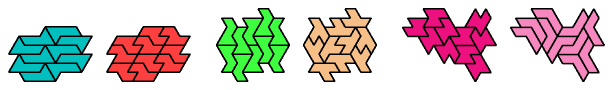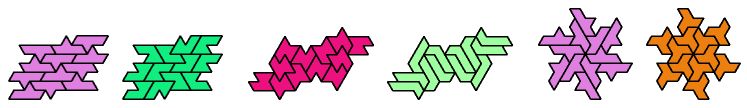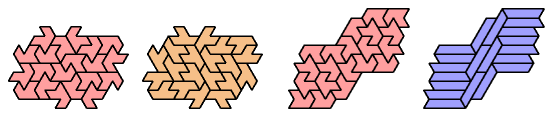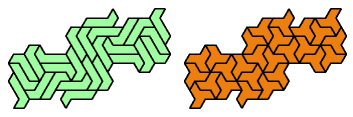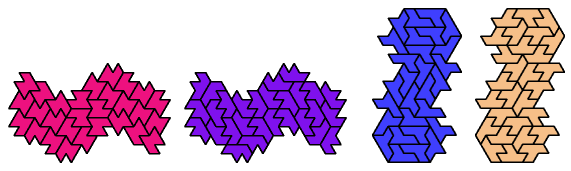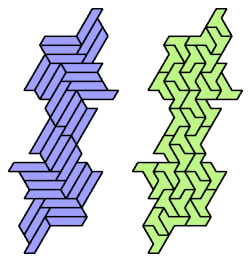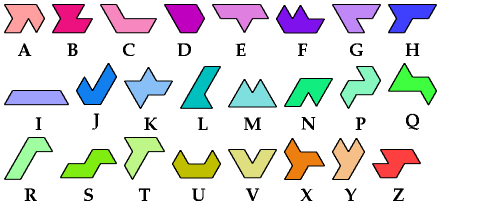
Dr. Erich Friedman's Math Magic for September 2004 shows compatibility figures for polyiamonds up to order 6 (and many other polyforms). Here I present the smallest known compatibility figures for heptiamonds.

  • Minimal Solutions
  • Holeless Variants

    • Nomenclature

    I adopt the nomenclature of K. Ishino's Heptiamonds Page.

    Minimal Solutions

    Summary

     ABCDEFGHIJKLMNPQRSTUVXYZ
    A*233232310323633322363663
    B2*362322262223183322??633
    C33*3323322?2624323222423
    D363*333622?3633323633663
    E2233*2322623663332333322
    F33232*26323363262623??23
    G223332*222622222232?31224
    H3236262*262326223232?262
    I102222322*2?2929922444?22
    J362262262*36?32224322?22
    K22??2362?3*363636233?336
    L32233323263*22542332312?64
    M626666229?62*2?212186???62
    N3323632623322*33333?2?62
    P31843322292654?3*362633222
    Q333336229232233*2234?1862
    R23223223226312362*2233626
    S223326322423183222*346222
    T322632234332636323*618623
    U6?2333?24233??34346*2366
    V3?233?3?42?12?23?36182*663
    X66463?122??3???21862636*32
    Y6326222622366626222663*2
    Z33332342226422226236322*
     ABCDEFGHIJKLMNPQRSTUVXYZ

    2 Tiles

    3–4 Tiles

    6–66 Tiles

    Holeless Variants

    Below I show only solutions that are not shown above.

    Summary

    Green cells indicate solutions that are minimal even without the condition of holelessness.

     ABCDEFGHIJKLMNPQRSTUVXYZ
    A*293232320?2??336226636203
    B2*10?228222?22222?61222???84
    C910*3423623?2624323262426
    D3?3*?43622???33323643663
    E224?*2?22?24?126?423??1222
    F328242*2?32???4262924??23
    G2233?2*222?22222232???24
    H32662?2*2?2226224262?2282
    I202222322*2?2?214?22364??22
    J??32?22?2*????22216?43?22
    K22??2??2??*?6?64?24?????
    L?22?4?222??*22?2462????10
    M?26???22??62*2?2???????2
    N32223124262??22*3363??2??2
    P3?4362221426??3*462633222
    Q6633?622?242234*2244??102
    R21222422422?4?662*223?2628
    S2233293221626?3222*44?222
    T6226322636?42??6424*6?623
    U6?64?4?244????34346*?6?6
    V3?23?????3???23?????*??3
    X6?4612??2??????2?26266?*32
    Y208262222822????210222??3*2
    Z3463234222?1022228236322*
     ABCDEFGHIJKLMNPQRSTUVXYZ

    3 Tiles

    4 Tiles

    6 Tiles

    8 Tiles

    9 Tiles

    10 Tiles

    12 Tiles

    14 Tiles

    16 Tiles

    20 Tiles

    22 Tiles

    26 Tiles

    28 Tiles

    36 Tiles

     

    Last revised 2012-01-04.
    Back to     Pairwise Compatibility < Polyform Compatibility < Polyform Curiosities
    Col. George Sicherman [ HOME | MAIL ]