Polyhex Oddities
A polyhex oddity is a plane figure with binary
symmetry formed by joining an odd number of copies of a polyhex.
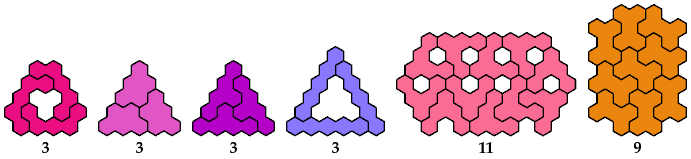
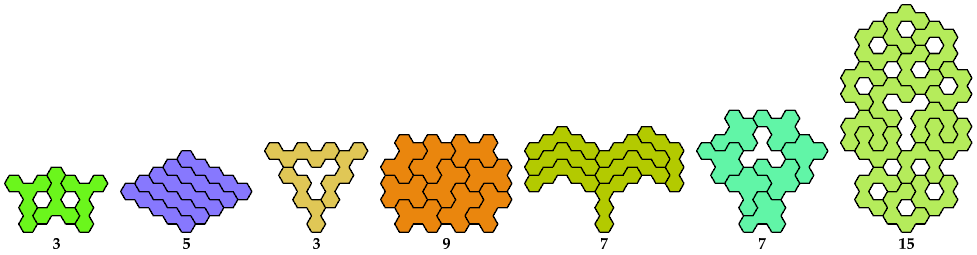
Here are the minimal known oddities for the trihexes,
tetrahexes, and pentahexes.
Please write if you find a smaller solution or solve an unsolved case.
For hexahexes, see
Hexahex Oddities.
Rowwise
Bilateral
| Columnwise
Bilateral
| Birotary | Double
Bilateral
| Ternary on Cell
Rowwise
Bilateral
| Ternary on Cell
Columnwise
Bilateral
| Ternary on Vertex
Rowwise
Bilateral
|
1

| 9
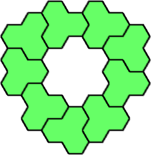
| 11

| 11

| 3
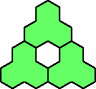
| 9
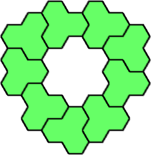
| 1

|
1

| 1

| 1

| 1

| 3
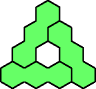
| 9
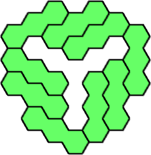
| 3

|
3

| 1

| 5
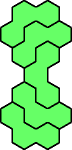
| 5

| 9

| 3
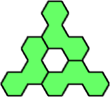
| 3

|
Holeless Variants
Ternary on Vertex, Rowwise Bilateral
 Mike Reid
proved that the O and S tetrahexes have no sexirotary oddities.
Mike Reid
proved that the O and S tetrahexes have no sexirotary oddities.
Rowwise
Bilateral
| Columnwise
Bilateral
| Birotary | Double
Bilateral | Sextuple
Rotary | Full |
1

| 1

| 1

| 1

| 9

| 9

|
3

| 3

| 3
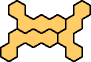
| 3

| 3

| 3

|
1

| 1

| 1

| 1

| None
| None
|
3
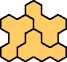
| 3

| 3

| 3
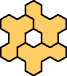
| 3

| 3

|
3
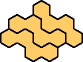
| 3

| 1

| 3

| None
| None
|
1

| 3

| 3

| 3

| 3

| 3

|
| None
| 1

| None
| None
| None
| None
|
Holeless Variants
Columnwise Bilateral
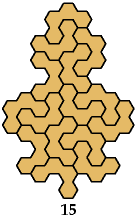
Double Bilateral
 Pentahexes are tricky, so I got help from
Mike Reid.
Click on the gray figures to expand them.
Pentahexes are tricky, so I got help from
Mike Reid.
Click on the gray figures to expand them.
[ Holeless Variants
| Composite Solutions
| Nontrivial Variants
| Mirror-Symmetric Tilings ]
| Rowwise Bilateral
| Columnwise Bilateral
| Birotary | Double
Bilateral | Sextuple
Rotary | Full |
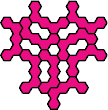
1

| 9

| 11

| 11

|
| |
1

| 9
|
|
|
| |
1

| 3

| 5

Mike Reid
| 5

Mike Reid
| 11

Mike Reid
| 11

Mike Reid
|
1

| 9

| 9

| 9

|
|
|
3

| 5
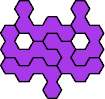
| 7

| 11

(after Mike Reid)
| 29

| 29
|
3

| 3

| 7
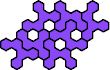
| 11

| 23

| 29

|
1

| 1

| 1

| 1

| 59
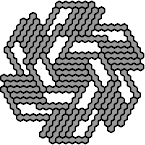
|
|
3

| 3

| 5
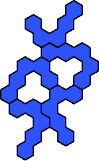
| 7

Mike Reid
| 29

|
|
3

| 3

| 5

Mike Reid
| 9

| 17

| 35

|
3

| 3

| 5
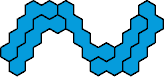
Mike Reid
| 9

Mike Reid
| 17

| 23

|
3

| 3

| 3

| 5

Mike Reid
| 17

| 29

|
3

| 3

| 5

| 7

| 11

Mike Reid
| 11

Mike Reid
|
5

| 1

| 11
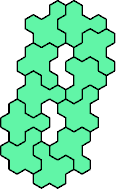
| 15

| 41

| 47

Mike Reid
|
3

| 5

| 7

| 11

| 23

| 35

|
7

| 3
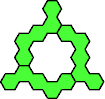
| 1
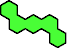
| 7

|
|
|
9
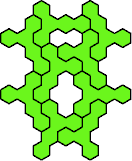
| 1

|
|
|
|
|
3

| 1

| 23

| 23

|
|
|
3

| 1

| 7

| 7

| 35

| 47

|
7

(squashed by Mike Reid)
| 1

| 9

| 9

| 53

| 53

|
1

| 1

| 1

| 1

| 101

|
|
3

| 5

| 7

| 9

| 17

| 17

|
5

| 5

| 7

| 15

| 17

| 17

|
Rowwise Bilateral
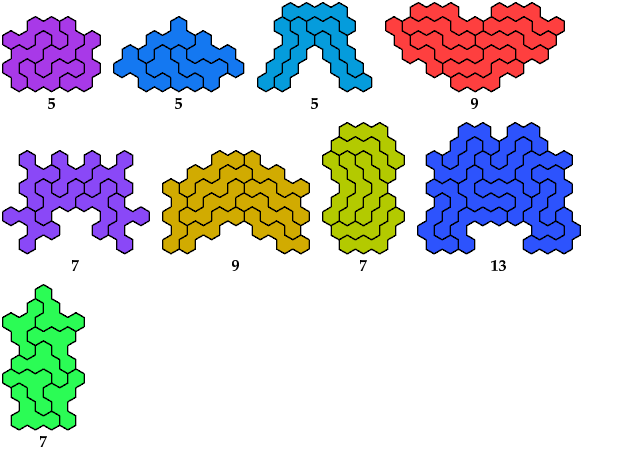
Columnwise Bilateral
Birotary

Double Bilateral
Sextuple Rotary
Full
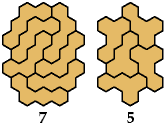
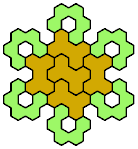
 Some pentahexes without oddities for certain symmetries
can be paired to form oddities.
Some pentahexes without oddities for certain symmetries
can be paired to form oddities.
Helmut Postl and Johann Schwenke found some of these
full-symmetric oddities.
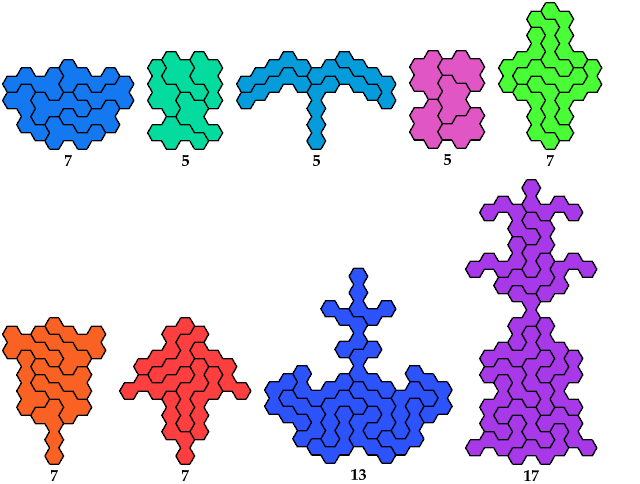
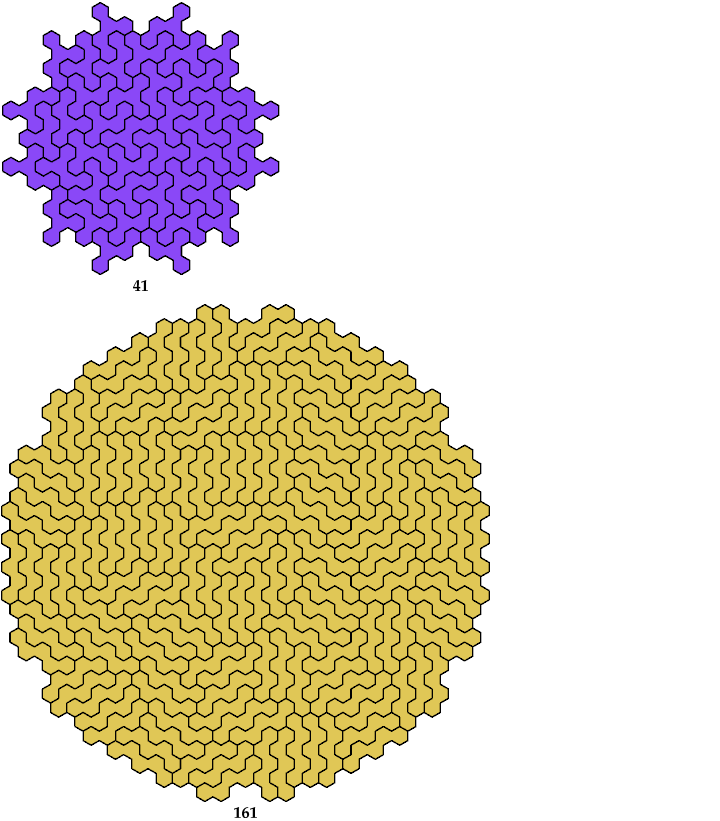
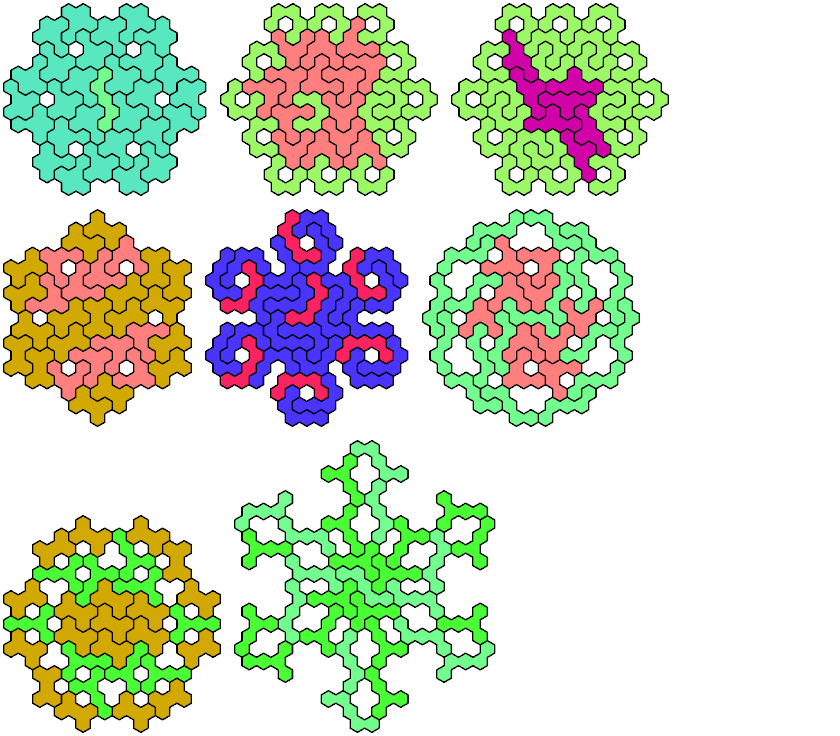
Birotary
9 Tiles
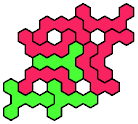
Double Bilateral
11 Tiles
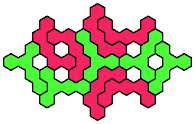
Sextuple Rotary
11 Tiles

17 Tiles

23 Tiles

47 Tiles
Full
11 Tiles
17 Tiles
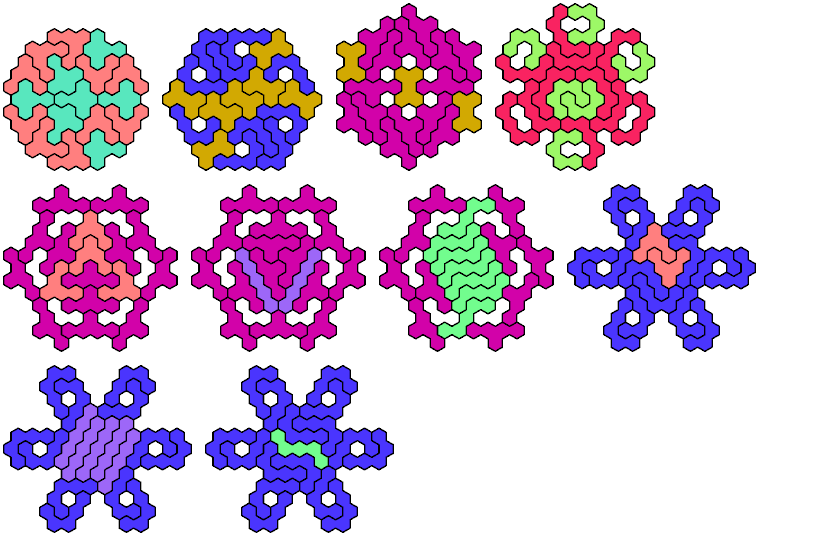
23 Tiles
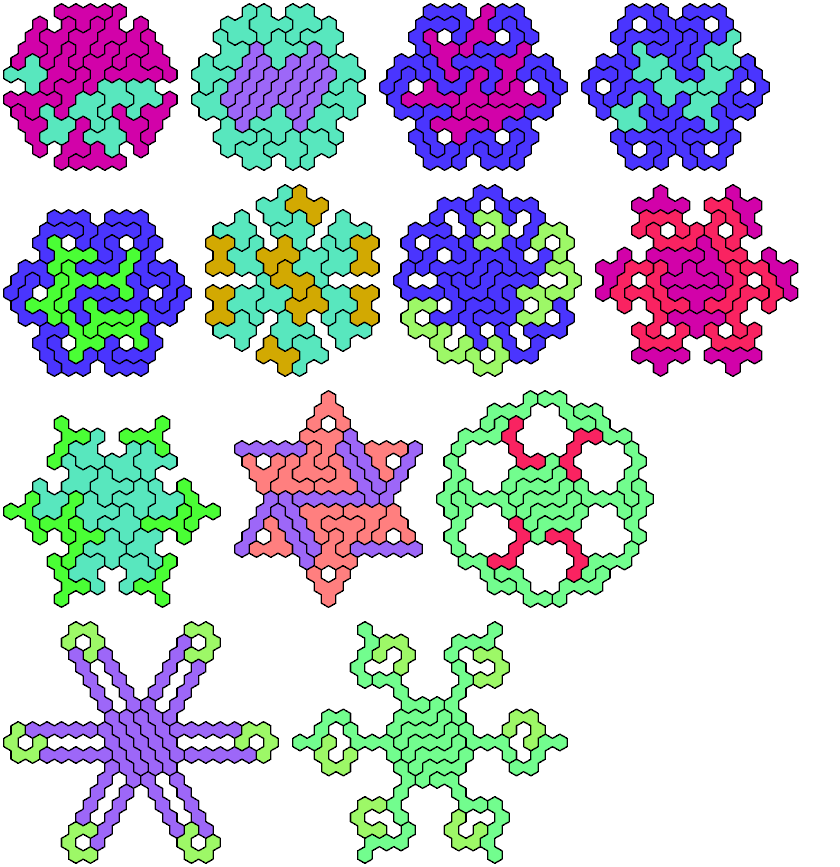
29 Tiles
35 Tiles

41 Tiles
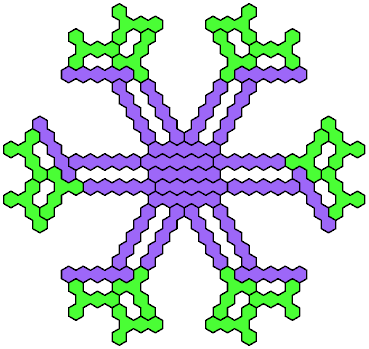
47 Tiles
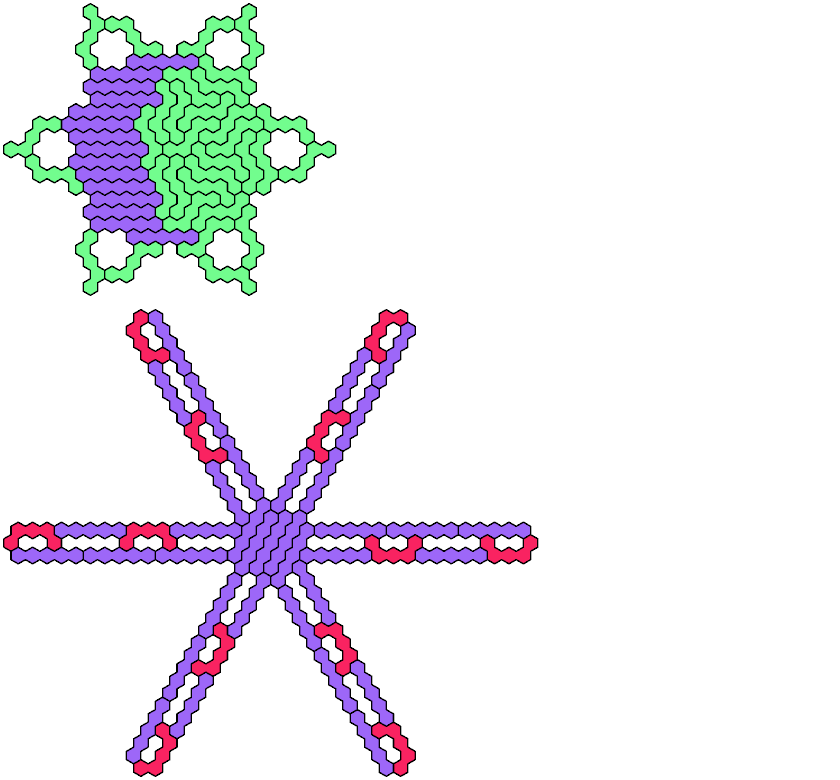
53 Tiles
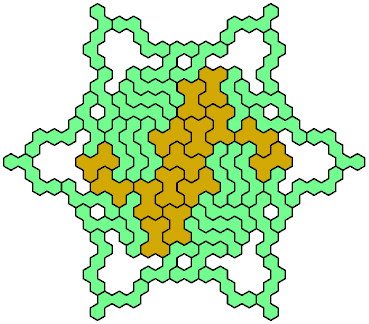
59 Tiles
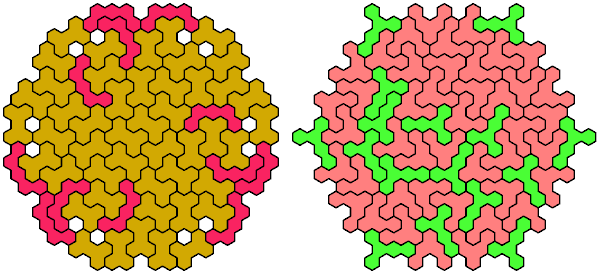
65 Tiles
 These tilings are irreducible and have more than one tile.
These tilings are irreducible and have more than one tile.
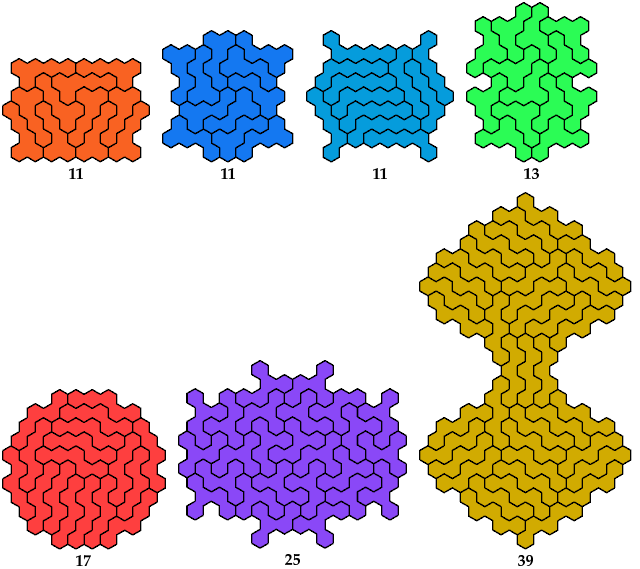
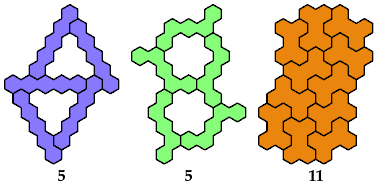
Rowwise Bilateral
Columnwise Bilateral
Birotary
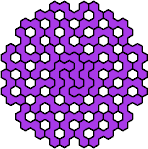
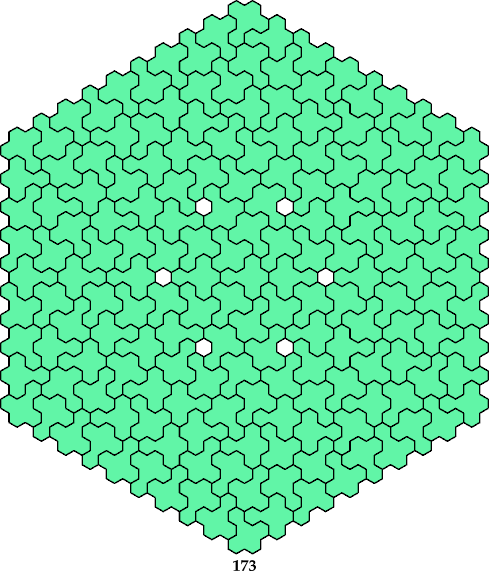
 Mike Reid found that this full-symmetry oddity for the Q pentahex
can be tiled with vertical mirror symmetry!
Mike Reid found that this full-symmetry oddity for the Q pentahex
can be tiled with vertical mirror symmetry!
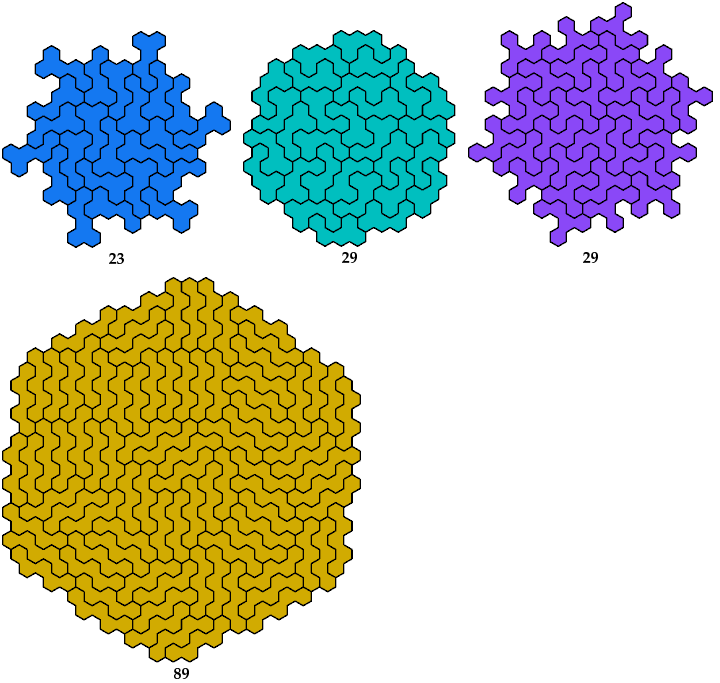
After Mike told me that a smaller solution probably existed,
I found this one:
Last revised 2023-01-23.
Back to
Polyform Oddities
< Polyform Curiosities
Col. George Sicherman
[ HOME
| MAIL
]








































































































