Two-Hexiamond Balanced Hexagons

Introduction

A hexiamond is a figure made of six equilateral triangles joined edge to edge. There are 12 such figures, not distinguishing reflections and rotations.

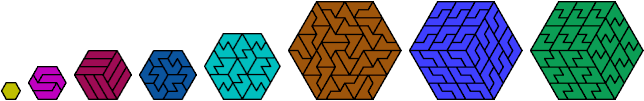
It has long been known that eight hexiamonds can tile regular hexagons:

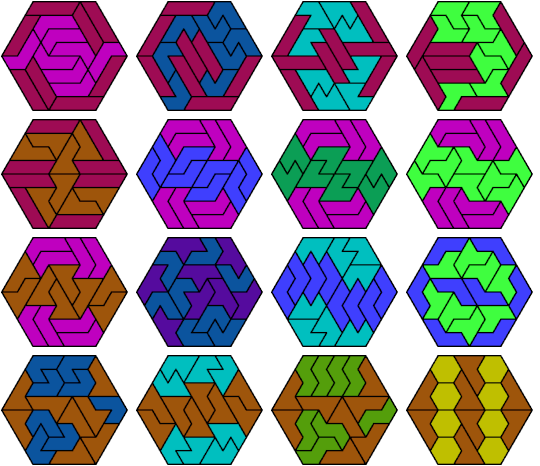
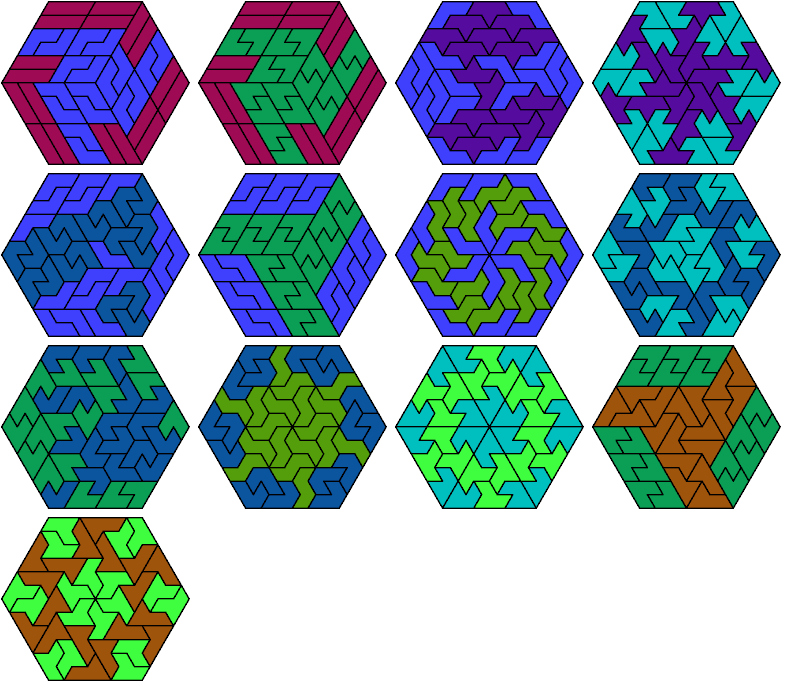
Here I study the related problem of tiling some regular hexagon with two hexiamonds, using the same number of copies of each. If you find a smaller solution or solve an unsolved case, please write.

For more general tilings with two hexiamonds, see the Poly Pages.

Nomenclature

Table

This table shows the smallest total number of hexiamonds known to be able to tile a regular hexagon in equal numbers.

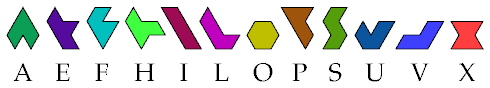
ILEVUFAHSOPX
I * 16 ? 36 16 16 36 16 144 ? 16 ?
L 16 * ? 16 4 4 16 16 324 ? 16 ?
E ? ? * 36 16 36 × × × 4 144 ×
V 36 16 36 * 36 16 36 16 36 144 4 4
U 16 4 16 36 * 36 36 4 36 ? 16 ?
F 16 4 36 16 36 * 4 36 144 ? 16 ?
A 36 16 × 36 36 4 * 144 324 ? 36 ?
H 16 16 × 16 4 36 144 * × ? 36 ×
S 144 324 × 36 36 144 324 × * × 16 ×
O ? ? 4 144 ? ? ? ? × * 16 ×
P 16 16 144 4 16 16 36 36 16 16 * 144
X ? ? × 4 ? ? ? × × × 144 *

Solutions

So far as I know, these solutions have minimal area. They are not necessarily uniquely minimal.

4 Tiles

16 Tiles

36 Tiles

144 Tiles

324 Tiles

Last revised 2012-06-29.

Back to Polyiamond and Polyming Tiling < Polyform Tiling < Polyform Curiosities
Col. George Sicherman [ HOME | MAIL ]