Minimal Incompatibility for Polyiamonds

Introduction

The compatibility problem is to find a figure that can be tiled with each of a set of polyforms. Here I show for each polyiamond of orders 1 through 7 the smallest known polyiamonds that are not compatible with it.

In some cases, incompatibility is probable but has not been proved by analysis or exhaustion. Proved cases are shown in red. Unproved cases are shown in blue.

Andris Cibulis first studied diamond compatibility and found the minimal polyiamond incompatible with the diamond.

See also Minimal Incompatibility for Polyominoes and Minimal Incompatibility for Polyhexes.

Solutions

Moniamond
	∞	None
Diamond
	14
Triamond
	12
Tetriamonds
	6			10
	5
Pentiamonds
	6		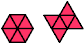	10
	6			4
Hexiamonds
	6			8
	4			6
	6			9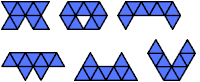
	6			8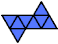
	6			4
	8			4
Heptiamonds
	6			6
	6			5
	6			4
	6			4
	6			6
	6			5
	5			6
	4		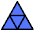	6
	6			6
	4			4
	4			4
	6			4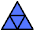

Last revised 2015-03-21.