Polyomino Rectification with Holes

Introduction

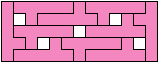
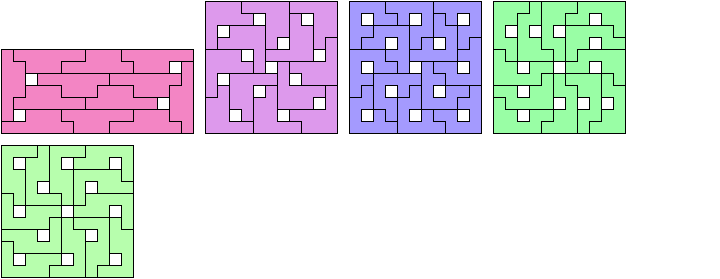
Here I show minimal tilings of rectangles, allowing single-cell holes in the interior of the rectangle. The holes may not touch, even at corners.

I omit polyominoes with fewer than 5 cells. When minimal rectangles for such polyominoes exist, they have no holes.

Thanks to Jenard Cabilao for suggesting allowing holes when tiling rectangles.

For rectification without holes, see Mike Reid's Rectifiable Polyomino Page.

Pentominoes

1 Tile

2 Tiles

8 Tiles

10 Tiles

Hexominoes

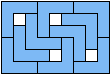
1 Tile

2 Tiles

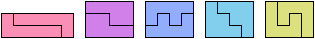
4 Tiles

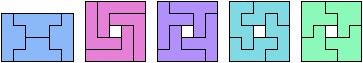
10 Tiles

16 Tiles

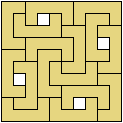
49 Tiles

Heptominoes

1 Tile

2 Tiles

6 Tiles

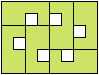
8 Tiles

10 Tiles

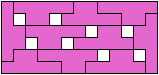
12 Tiles

16 Tiles

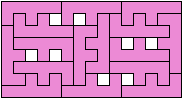
20 Tiles

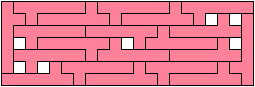
22 Tiles

24 Tiles

26 Tiles

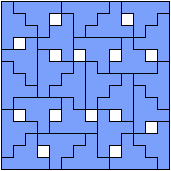
36 Tiles

Octominoes

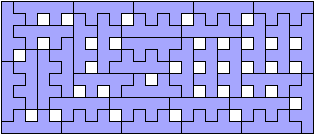
1 Tile

2 Tiles

4 Tiles

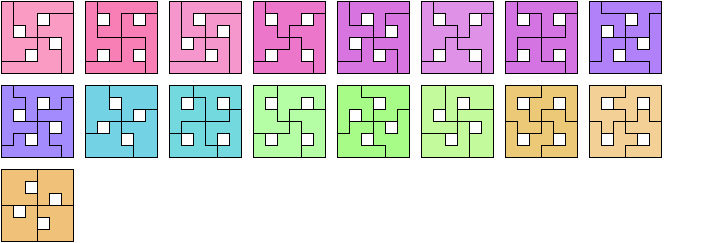
6 Tiles

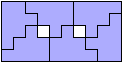
10 Tiles

11 Tiles

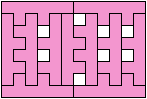
12 Tiles

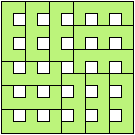
23 Tiles

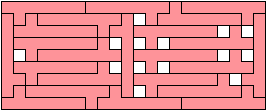
24 Tiles

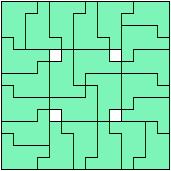
26 Tiles

30 Tiles

Enneominoes

1 Tile

2 Tiles

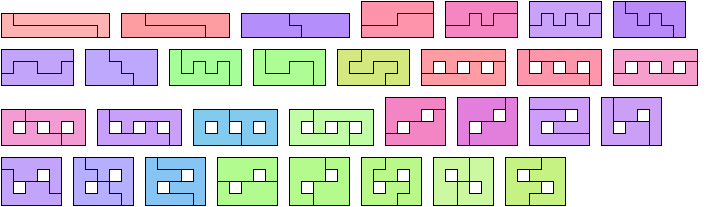
4 Tiles

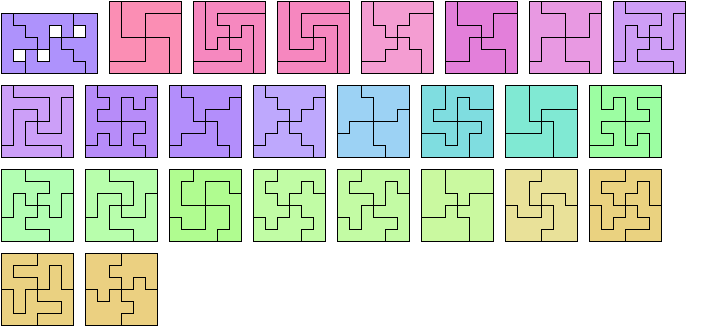
8 Tiles

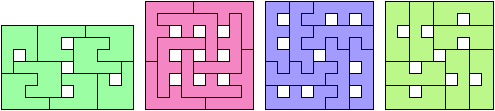
12 Tiles

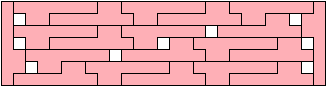
20 Tiles

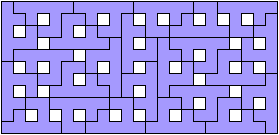
24 Tiles

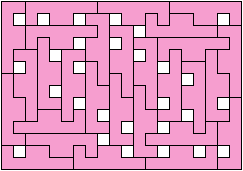
25 Tiles

28 Tiles

Last revised 2023-09-17.