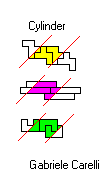
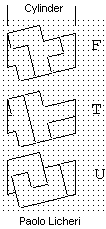
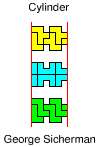
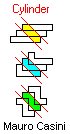
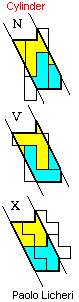
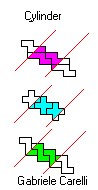
We are searching for the 220 shapes that can be covered by three different pentominoes at least. We'll give precedence to the solutions on the finite plane with the smallest surface. If there aren't solutions on the plane, we'll accept solutions on cylindrical surface or on Moebius strip. Solutions on torus are not interesting because eac pentomino cover a torus. 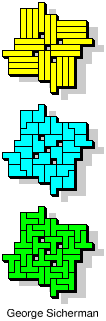If you have better solutions, please write to George Sicherman HERE.

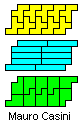
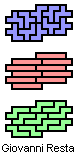
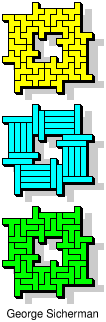
F5I5L5	F5I5P5	F5I5N5	F5I5T5	F5I5U5
F5I5V5	F5I5W5	F5I5X5	F5I5Y5	F5I5Z5
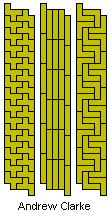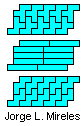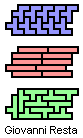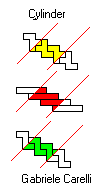F5L5P5	F5L5N5	F5L5T5	F5L5U5	F5L5V5
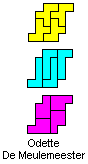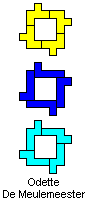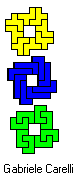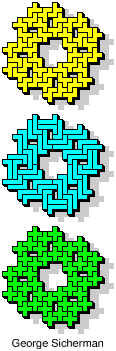
F5L5W5	F5L5X5	F5L5Y5	F5L5Z5	F5P5N5
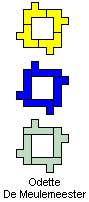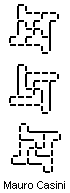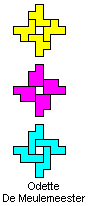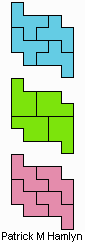
F5P5T5	F5P5U5	F5P5V5	F5P5W5	F5P5X5
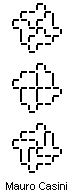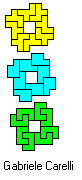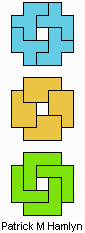F5P5Y5	F5P5Z5	F5N5T5	F5N5U5	F5N5V5
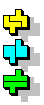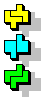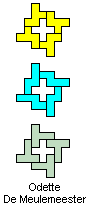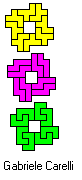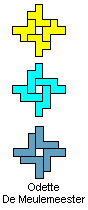F5N5W5	F5N5X5	F5N5Y5	F5N5Z5	F5T5U5
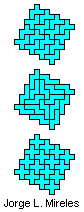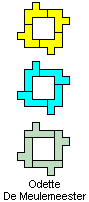F5T5V5	F5T5W5	F5T5X5	F5T5Y5	F5T5Z5
F5U5V5	F5U5W5	F5U5X5	F5U5Y5	F5U5Z5
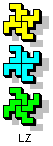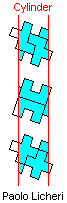F5V5W5	F5V5X5	F5V5Y5	F5V5Z5	F5W5X5
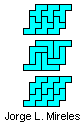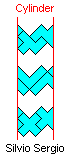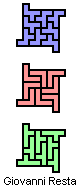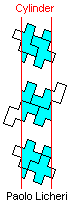F5W5Y5	F5W5Z5	F5X5Y5	F5X5Z5	F5Y5Z5
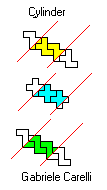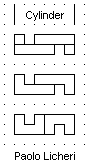
I5L5P5	I5L5N5	I5L5T5	I5L5U5	I5L5V5
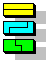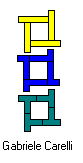I5L5W5	I5L5X5	I5L5Y5	I5L5Z5	I5P5N5
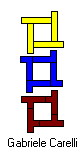I5P5T5	I5P5U5	I5P5V5	I5P5W5	I5P5X5
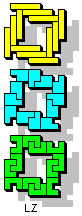I5P5Y5	I5P5Z5	I5N5T5	I5N5U5	I5N5V5
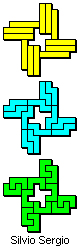I5N5W5	I5N5X5	I5N5Y5	I5N5Z5	I5T5U5
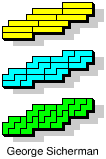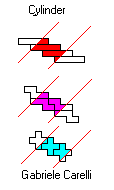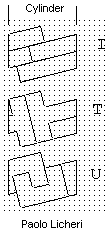I5T5V5	I5T5W5	I5T5X5	I5T5Y5	I5T5Z5
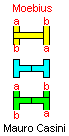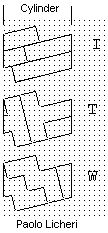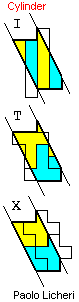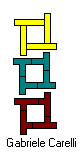I5U5V5	I5U5W5	I5U5X5	I5U5Y5	I5U5Z5
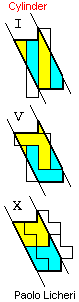
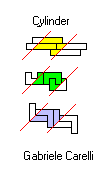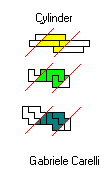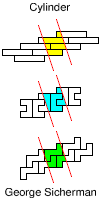I5V5W5	I5V5X5	I5V5Y5	I5V5Z5	I5W5X5
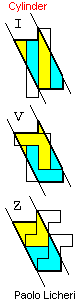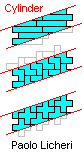I5W5Y5	I5W5Z5	I5X5Y5	I5X5Z5	I5Y5Z5
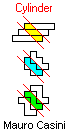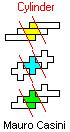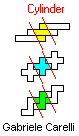L5P5N5	L5P5T5	L5P5U5	L5P5V5	L5P5W5
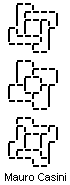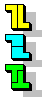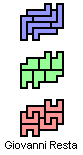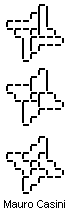L5P5X5	L5P5Y5	L5P5Z5	L5N5T5	L5N5U5
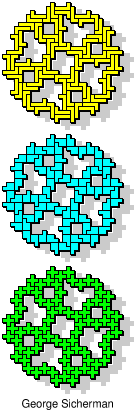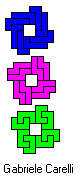L5N5V5	L5N5W5	L5N5X5	L5N5Y5	L5N5Z5
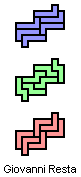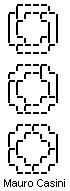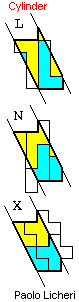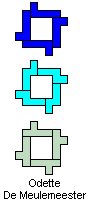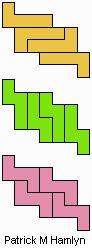L5T5U5	L5T5V5	L5T5W5	L5T5X5	L5T5Y5
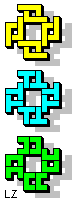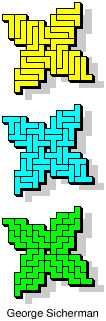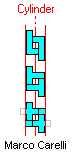L5T5Z5	L5U5V5	L5U5W5	L5U5X5	L5U5Y5
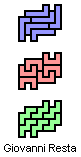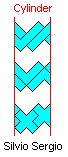
L5U5Z5	L5V5W5	L5V5X5	L5V5Y5	L5V5Z5
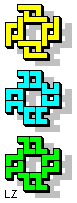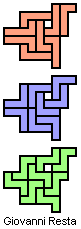L5W5X5	L5W5Y5	L5W5Z5	L5X5Y5	L5X5Z5
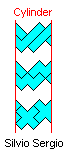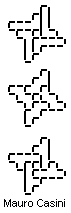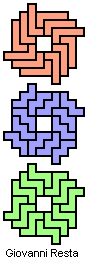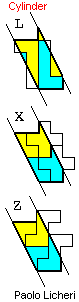L5Y5Z5	P5N5T5	P5N5U5	P5N5V5	P5N5W5
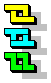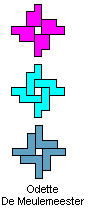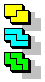P5N5X5	P5N5Y5	P5N5Z5	P5T5U5	P5T5V5
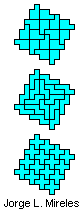P5T5W5	P5T5X5	P5T5Y5	P5T5Z5	P5U5V5
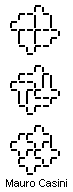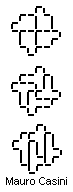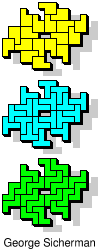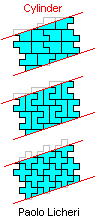
P5U5W5	P5U5X5	P5U5Y5	P5U5Z5	P5V5W5
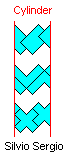
P5V5X5	P5V5Y5	P5V5Z5	P5W5X5	P5W5Y5
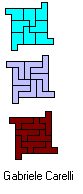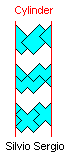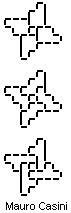P5W5Z5	P5X5Y5	P5X5Z5	P5Y5Z5	N5T5U5
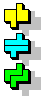N5T5V5	N5T5W5	N5T5X5	N5T5Y5	N5T5Z5
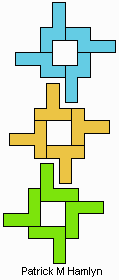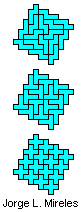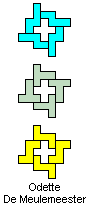N5U5V5	N5U5W5	N5U5X5	N5U5Y5	N5U5Z5
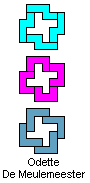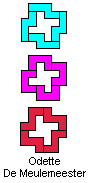N5V5W5	N5V5X5	N5V5Y5	N5V5Z5	N5W5X5
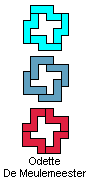N5W5Y5	N5W5Z5	N5X5Y5	N5X5Z5	N5Y5Z5
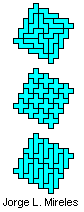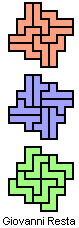T5U5V5	T5U5W5	T5U5X5	T5U5Y5	T5U5Z5
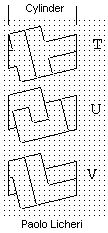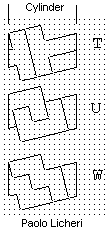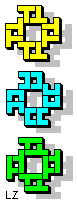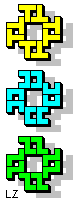T5V5W5		T5V5X5	T5V5Y5	T5V5Z5
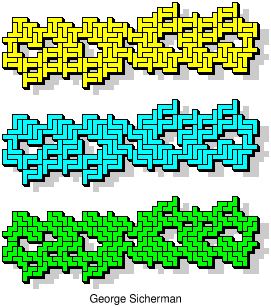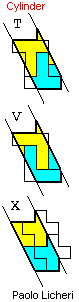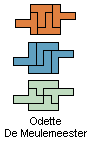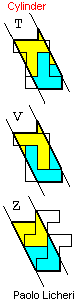T5W5X5	T5W5Y5	T5W5Z5	T5X5Y5	T5X5Z5
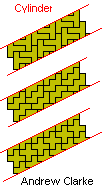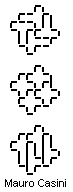T5Y5Z5	U5V5W5	U5V5X5	U5V5Y5	U5V5Z5
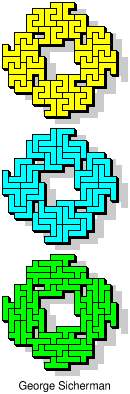U5W5X5	U5W5Y5	U5W5Z5	U5X5Y5	U5X5Z5
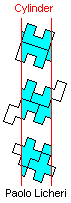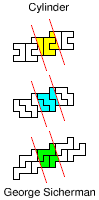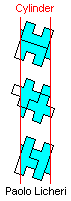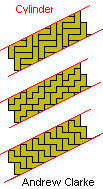
U5Y5Z5	V5W5X5	V5W5Y5	V5W5Z5	V5X5Y5
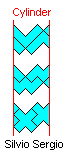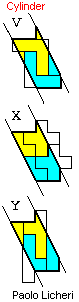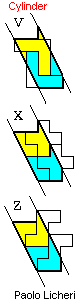
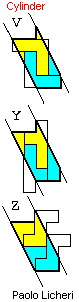
V5X5Z5	V5Y5Z5	W5X5Y5	W5X5Z5	W5Y5Z5
X5Y5Z5

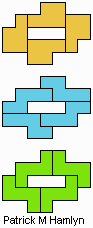
Here below we are searching for the shape of minimal area that can be covered by the maximal number of different pentominoes.

PLANE

CYLINDER

TORUS

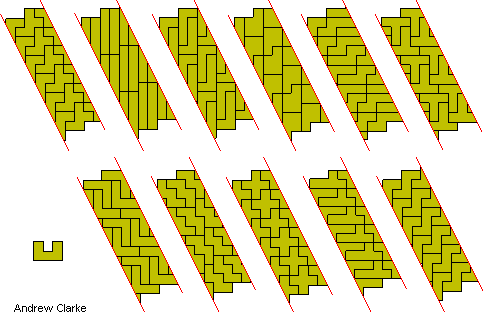
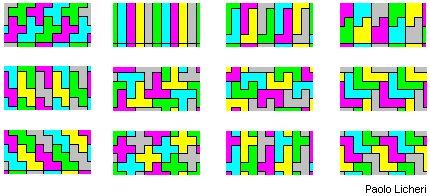
Notice:

1) I just find this solutions on our website:
http://pentomino.classy.be/conmeerlingen.html
so it isn't really of me. The most I got from Patrick Hamlyn whose found this quadruples by Peter Essers program and others are from Aad van de Wetering. [Odette De Meulemeester]

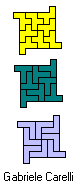
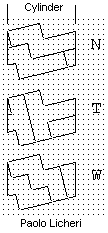
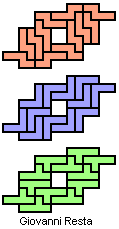
2) I can't attribute the exact paternity of these solutions. The author name on each drawing is of who signals the solution in this game. [Livio Zucca]

3) I think that from 66 pentomino-pentomino solutions (if they are minimal) then can be derived some for the 220 pentomino-pentomino-pentomino (minimal also). [Jorge L. Mireles]

See also:

• Pento-tro-dominoes
• Pento-tetro-trominoes
• Tetrominoes Challenge

LINKS:
Holeless Triple Pentominoes
Quadruple Pentominoes
Pentomino Odd Triples
Multiple Compatibility for Polyominoes
Triple Hexiamonds
Visit the wonderful site of Jorge Luis Mireles (archived).
The pages of Giovanni Resta.