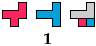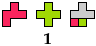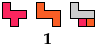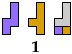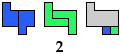Cell Shifts for Pentomino Pairs
Introduction
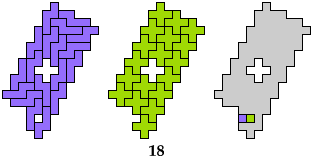
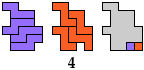
One figure can be tiled with one pentomino, and
another figure can be tiled with another pentomino.
The figures differ in only one cell.
How near can the unmatched cells lie?
Here I show minimal shift vectors for pairs of pentominoes.
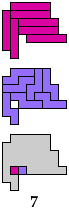
The only unsolved cases are the pairs not known to be compatible:
I+X, U+X, V+X, W+X, and X+Z.
(For compatibility results see
Pentomino Compatibility.)
If you find a smaller solution for any of these pairs, please let me know.
Table
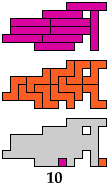
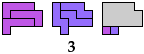
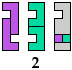
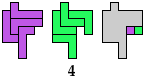
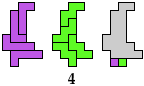
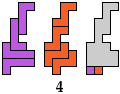
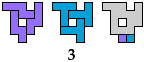
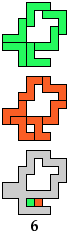
Green cells denote shifts of three units.
Orange cells denote shifts of five units.
| F | I | L | N | P | T | U | V | W | X | Y | Z |
|---|
| F
| *
| 9
| 2
| 2
| 2
| 1
| 4
| 4
| 1
| 1
| 2
| 1
|
|---|
| I
| 9
| *
| 4
| 7
| 2
| 16
| 10
| 6
| 10
| ×
| 2
| 10
|
|---|
| L
| 2
| 4
| *
| 3
| 2
| 3
| 2
| 4
| 4
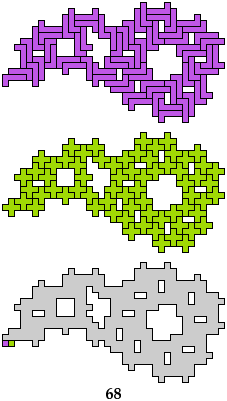
| 68
| 1
| 4
|
|---|
| N
| 2
| 7
| 3
| *
| 2
| 3
| 2
| 3
| 3
| 18
| 1
| 4
|
|---|
| P
| 2
| 2
| 2
| 2
| *
| 2
| 1
| 2
| 2
| 4
| 2
| 2
|
|---|
| T
| 1
| 16
| 3
| 3
| 2
| *
| 4
| 1
| 14
| 1
| 2
| 4
|
|---|
| U
| 4
| 10
| 2
| 2
| 1
| 4
| *
| 2
| 4
| ×
| 2
| 4
|
|---|
| V
| 4
| 6
| 4
| 3
| 2
| 1
| 2
| *
| 8
| ×
| 2
| 6
|
|---|
| W
| 1
| 10
| 4
| 3
| 2
| 14
| 4
| 8
| *
| ×
| 2
| 7
|
|---|
| X
| 1
| ×
| 68
| 18
| 4
| 1
| ×
| ×
| ×
| *
| 2
| ×
|
|---|
| Y
| 2
| 2
| 1
| 1
| 2
| 2
| 2
| 2
| 2
| 2
| *
| 2
|
|---|
| Z
| 1
| 10
| 4
| 4
| 2
| 4
| 4
| 6
| 7
| ×
| 2
| *
|
|---|
Solutions
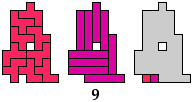
| 5F+5I | 5F+5L | 5F+5N | 5F+5P | 5F+5T | 5F+5U
|
 |
 |
 |
 |
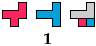 |
 |
| 5F+5V | 5F+5W | 5F+5X | 5F+5Y | 5F+5Z | 5I+5L |
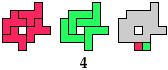 |
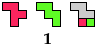 |
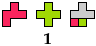 |
 |
 |
 |
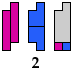
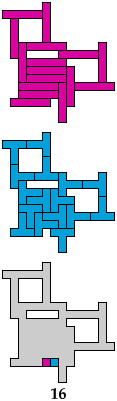
| 5I+5N | 5I+5P | 5I+5T | 5I+5U | 5I+5V | 5I+5W |
| 
| 
| 
| 
| 
|
| 5I+5X | 5I+5Y | 5I+5Z | 5L+5N | 5L+5P | 5L+5T |
 |
 |
 |
 |
 |
 |
| 5L+5U | 5L+5V | 5L+5W | 5L+5X | 5L+5Y | 5L+5Z |
| 
| 
| 
| 
| 
|
| 5N+5P | 5N+5T | 5N+5U | 5N+5V | 5N+5W | 5N+5X |
 |
 |
 |
 |
 |
 |
| 5N+5Y | 5N+5Z | 5P+5T | 5P+5U | 5P+5V | 5P+5W |
 |
 |
 |
 |
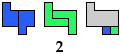 |
 |
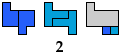
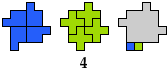
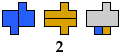
| 5P+5X | 5P+5Y | 5P+5Z | 5T+5U | 5T+5V | 5T+5W |
 |
 |
 |
 |
 |
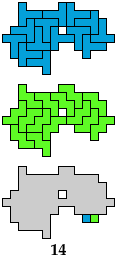 |
| 5T+5X | 5T+5Y | 5T+5Z | 5U+5V | 5U+5W | 5U+5X |

| 
| 
| 
| 
| 
|
| 5U+5Y | 5U+5Z | 5V+5W | 5V+5X | 5V+5Y | 5V+5Z |

| 
| 
| 
| 
| 
|
| 5W+5X | 5W+5Y | 5W+5Z | 5X+5Y | 5X+5Z | 5Y+5Z |
 |
 |
 |
 |
 |
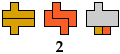 |
Last revised 2016-04-30.
Back to Polyform Cell Shifting
<
Polyform Compatibility
<
Polyform Curiosities.
Col. George Sicherman
[ HOME
| MAIL
]