Pentomino Compatibility

Introduction

A pentomino is a figure made of five squares joined edge to edge. There are 12 such figures, not distinguishing reflections and rotations. They were first enumerated and studied by Solomon Golomb.

The compatibility problem is to find a figure that can be tiled with each of a set of polyforms. Polyomino compatibility has been widely studied since 1992, when Pablo Coll first posed and studied the problem for pentominoes. Two well-known websites, Poly2ominoes by Jorge Mireles and Polypolyominoes by Giovanni Resta, present the results of their authors' systematic searches for compatibility figures. Mireles's site includes solutions by other researchers, especially Mike Reid. So far as I know, polyomino compatibility has not been treated in print since Golomb first raised the issue in 1981, except in Issue 3 of Rodolfo Marcelo Kurchan's periodical Puzzle Fun (1995), and in a series of articles called Polyomino Number Theory, written by Andris Cibulis, Andy Liu, Bob Wainwright, Uldis Barbans, and Gilbert Lee from 2002 to 2005.

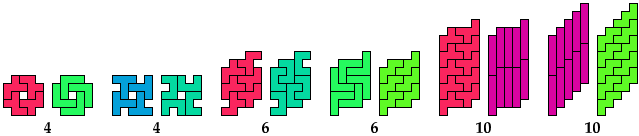
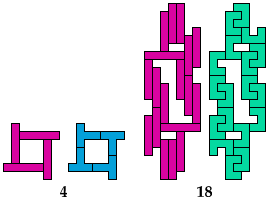
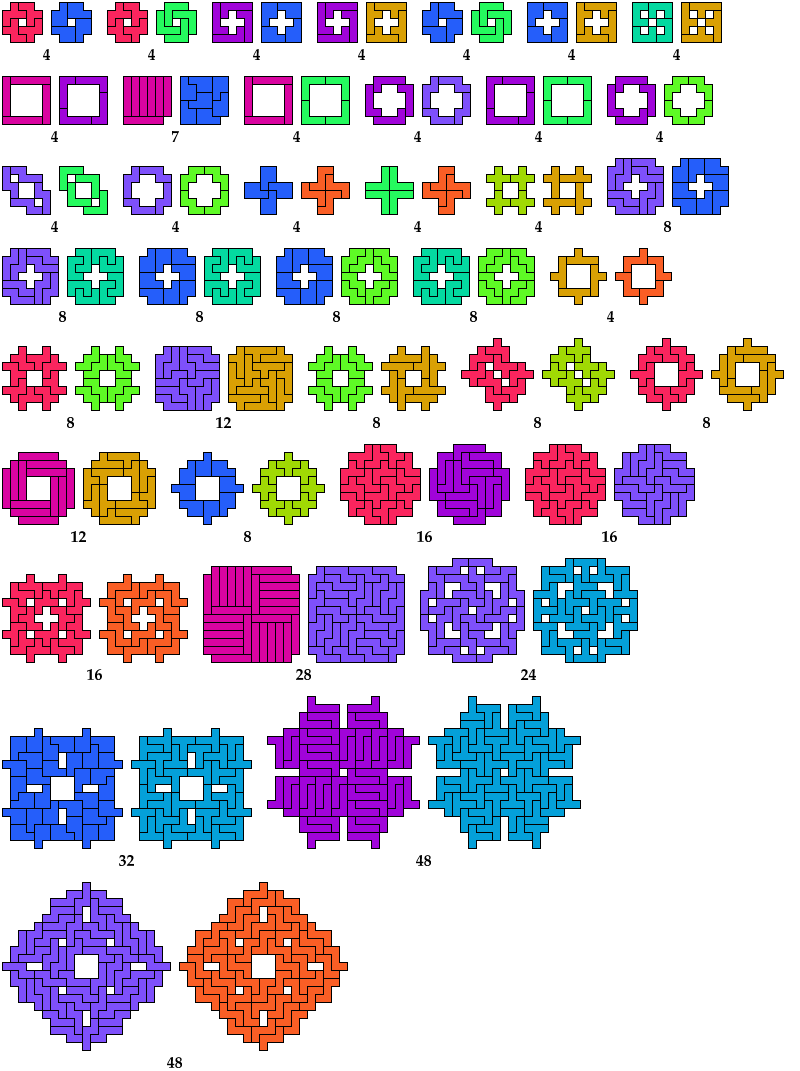
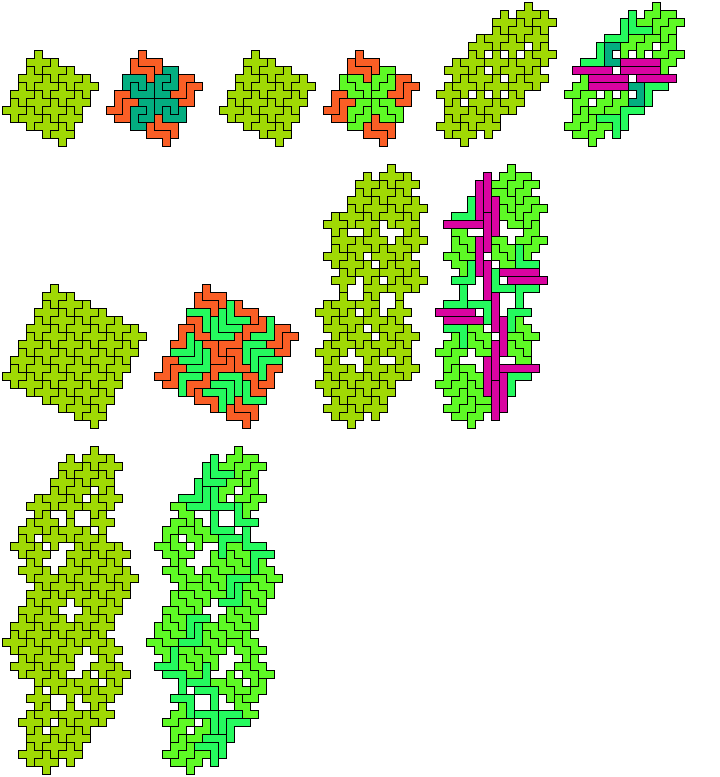
The websites and the articles present a wealth of polyomino compatibilities. They do not show all current results. I do so below, for pentomino-pentomino compatibility only. I am not prepared to maintain a current catalogue of results for other kinds of pairs of polyominoes, or for larger sets such as the pentomino triples that Livio Zucca has collected here.

I also show holeless variants. So far as I know, these have been covered before only in Puzzle Fun.

For compatibility figures with an odd number of tiles, see Livio Zucca's Pentomino Odd Pairs. For Galvagni compatibility (self-compatibility), see Galvagni Figures & Reid Figures for Pentominoes.

  • Basic Solutions
  • Thin Solutions
  • Diagonally Symmetric Solutions
  • Hybrid Solutions
  • Holeless Variants

    • Basic Solutions

    Table

    This table shows the smallest number of tiles known to suffice to construct a figure tilable by both pentominoes. The names used for the pentominoes are Golomb's original names.

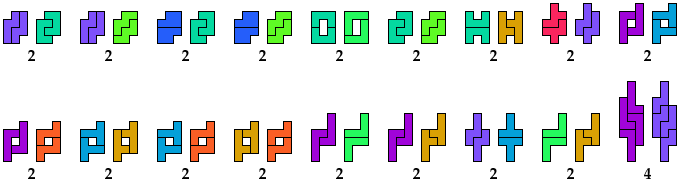
     FILNPTUVWXYZ
    F*102222442222
    I10*222412410×220
    L22*4222224422
    N224*222221622
    P2222*2222422
    T24222*4214422
    U4122224*22×24
    V4422222*6×24
    W2102221426*?24
    X2×441644××?*2?
    Y2222222222*2
    Z2202222444?2*

    Solutions

    So far as I know, these solutions are minimal. They are not necessarily uniquely minimal.

    2 Tiles

    4 Tiles

    6 Tiles

    10 Tiles

    12 Tiles

    14 Tiles

    16 Tiles

    20 Tiles

    44 Tiles

    Thin Solutions

    The thinnest compatibility figure for a pair of pentominoes is not always that with the smallest area. Here are the thinnest known solutions for pairs of pentominoes.

    Width 2

    Width 3

    Width 4

    Width 5

    Width 6

    Width 7

    Width 8

    Width 9

    Width 12

    Width 20

    Diagonally Symmetric Solutions

    If a solution has diagonal symmetry, four copies of it can be arranged to make a solution with square symmetry. However, diagonally symmetric solutions are hard to find.

    Hybrid Solutions

    The X pentomino is not compatible with any of the pentominoes I, U, V, W, and Z. However, it is compatible with some mixtures of them:

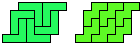
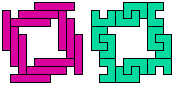
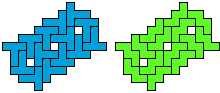
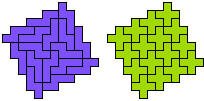
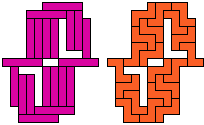
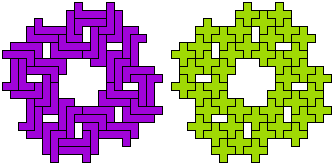
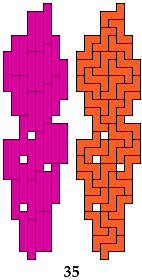
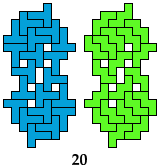
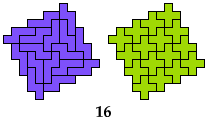
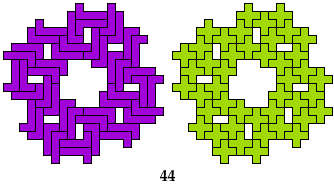
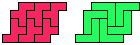
    Are there any other hybrid solutions?

     

    Holeless Variants

    Table

    The green figures represent holeless tilings that are minimal even without the condition of holelessness.

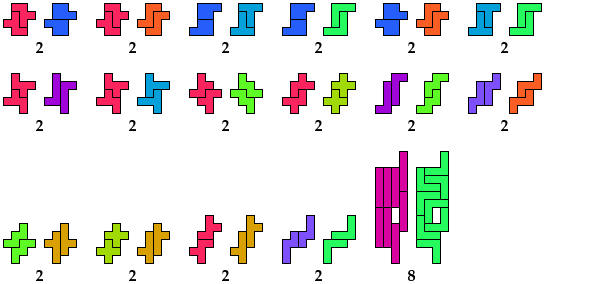
     FILNPTUVWXYZ
    F*102222462222
    I10*22232?1010×2?
    L22*422222×22
    N224*222221622
    P2222*2222422
    T232222*?2164230
    U4?222?*?2×2?
    V6102222?*6×24
    W2102221626*?210
    X2××1644××?*2?
    Y2222222222*2
    Z2?22230?410?2*

    Solutions

    I omit solutions that are the same as in Basic Solutions above. So far as I know, the following solutions are minimal. They are not necessarily uniquely minimal.

    6 Tiles

    10 Tiles

    16 Tiles

    30 Tiles

    32 Tiles

     

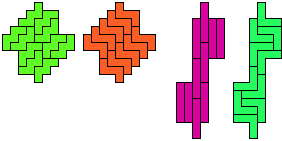
    Last revised 2022-04-17.

    Back to Pairwise Compatibility < Polyform Compatibility < Polyform Curiosities
    Col. George Sicherman [ HOME | MAIL ]