Polyhex Tetrads

A tetrad is a plane figure made of four congruent shapes, joined so that each shares a boundary with each.

The smallest polyhex tetrads use tetrahexes. The first, by Scott Kim, is from Gardner's book.

Symmetric Tiles

The smallest tetrad for a polyhex with birotary symmetry uses 6-hexes:

The smallest tetrads for polyhexes with vertical mirror symmetry use 7-hexes:

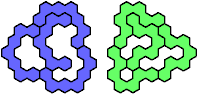
The smallest tetrads for polyhexes with birotary symmetry around a cell use 9-hexes:

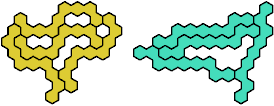
The smallest tetrad for polyhexes with ternary symmetry uses 9-hexes:

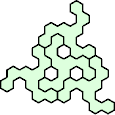
The smallest tetrad for polyhexes with ternary symmetry about a cell uses 13-hexes:

The smallest holeless tetrad for symmetric polyhexes uses 9-hexes:

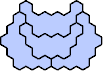
Last revised 2018-11-14.

Back to Polyform Tetrads < Polyform Curiosities
Col. George Sicherman [ HOME | MAIL ]