Pentacube Oddities with Full Symmetry

Introduction

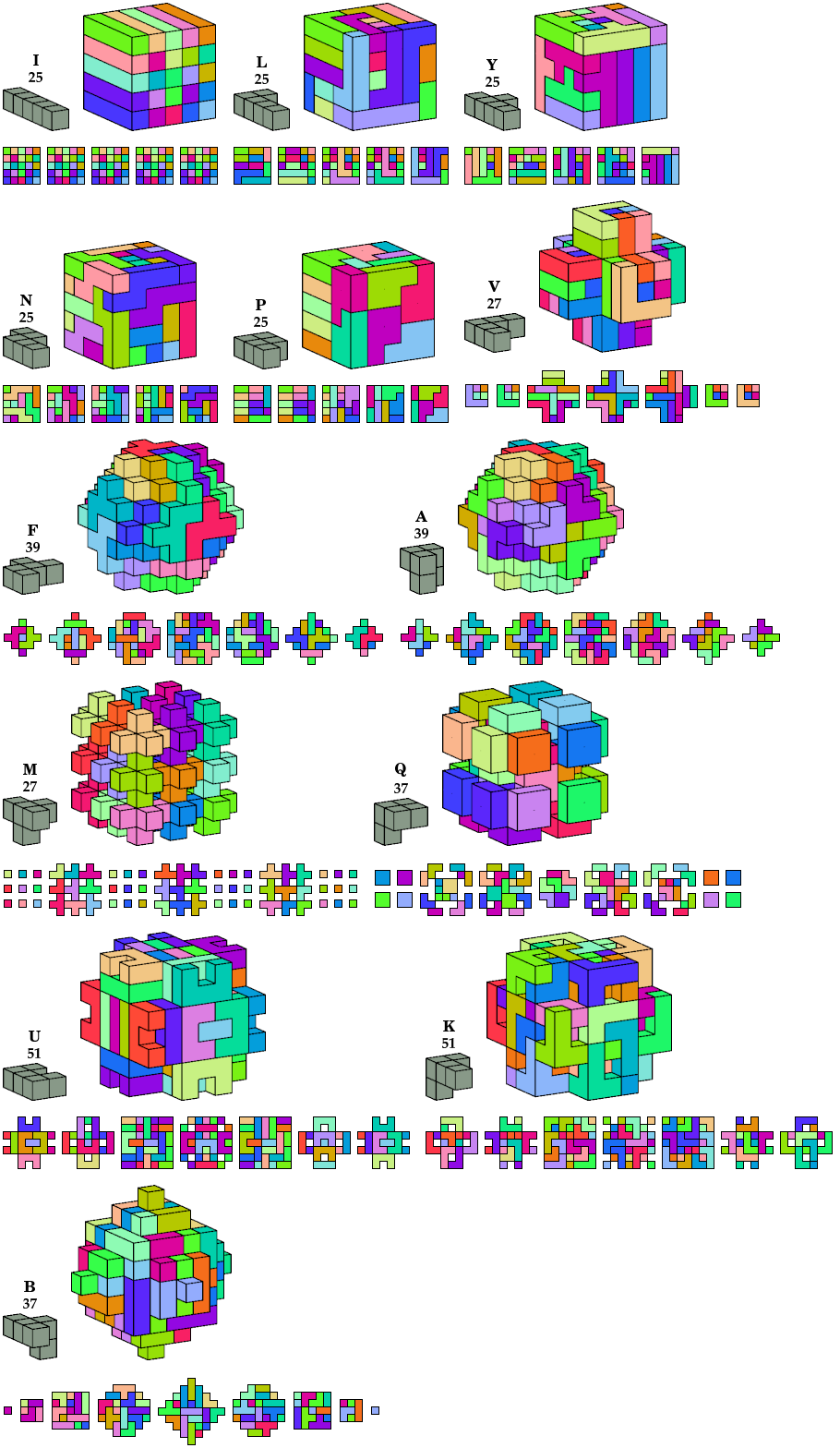
Polycubes have 33 symmetry classes (including asymmetry), and 31 of them have even order. That is too many to show here. Instead I show only oddities with full cubic symmetry.

For other classes of symmetry, see:

In all pictures, the cross-sections are shown from back to front.

Thanks to Jaap Scherphuis for pointing out an error in one of my chiral tilings.

Full Symmetry

The 5×5×5 cubes are due to Torsten Sillke.

Achiral Pentacubes

The oddity for the B pentacube can also be tiled by the Q pentacube.

Pentacube	Boxes	Shape	Tiles
	3×12×25 3×13×25 6×8×25 7×8×25	25×25×25 cube	3125
	5×5×14 5×5×19	3D cross with arms 5×5×14	445
	5×9×25 5×16×25	25×25×25 cube	3125

Unsolved

Chiral, Disallowing Reflection

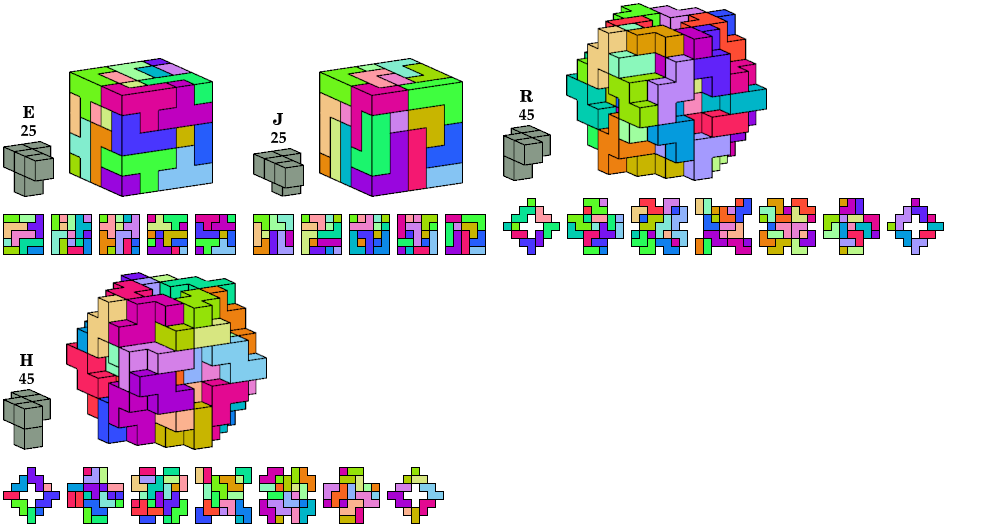
Pentacube	Boxes	Cube	Tiles
	5×9×15 6×6×15 6×9×15	15×15×15	675

Unsolved

Chiral, Allowing Reflection

Pentacube	Boxes	Cube	Tiles
	3×5×9 5×5×6	15×15×15	675

Last revised 2024-03-07.