

A reptiling of a polyform is a dissection of it into two or more equal pieces that are similar to the original polyform. An irreptiling of a polyform is a dissection of it into pieces that are all similar to the original polyform, but not necessarily of equal size. Some shapes have irreptilings and no reptilings.
Here I omit polyiamonds that are similar to polyiamonds with fewer cells, and I show only tilings with the fewest possible pieces. If you find a tiling with fewer pieces, or solve an unsolved case, please write.
Dr. Karl Scherer coined the term irreptile and was the first to study irreptiles. Many of these tilings are taken from his book A Puzzling Journey to the Reptiles and Related Animals.
See also Polydrafter Irreptiling. For irreptilings of polyforms other than polyiamonds, see Erich Friedman's Math Magic for October 2002.

|  |
| 4 |

|  |
| 4 |

|  |
| 4 |

|  |
| 4 |

|  |
| 8 | |

| 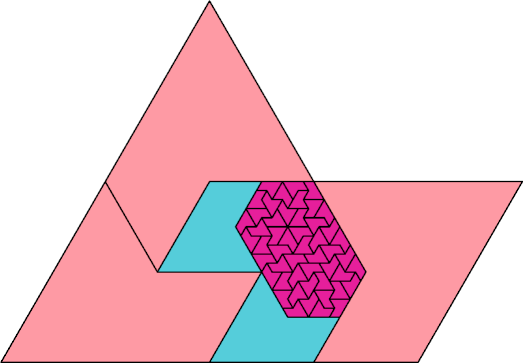
Andrew Bayly |
| 55 |

| 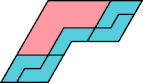
Karl Scherer |
| 6 | |

| 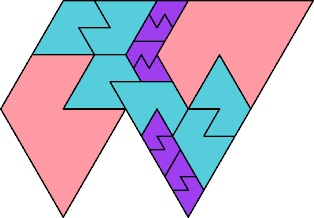
Karl Scherer |
| 16 | |

| 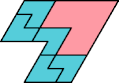
Karl Scherer |
| 6 | |

|  |
| 4 |

| 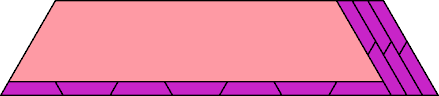 |
| 14 |

|  |
| 4 | |

|  |
| 4 | |

| 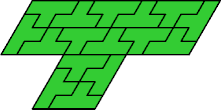 |
| 16 | |

|  |
| 4 | |

| 
Karl Scherer |
| 10 | |

|  |
| 9 | |

| 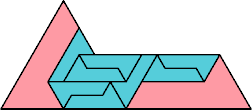
Karl Scherer |
| 10 |

|  |
| 18 | |

| 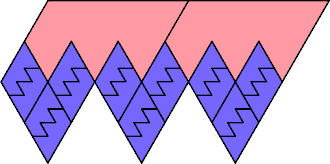 |
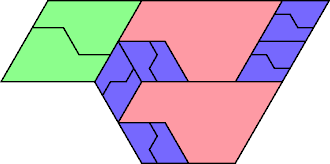
| 20 | |

| 
Karl Scherer |
| 14 |

|  |
| 4 | |

| 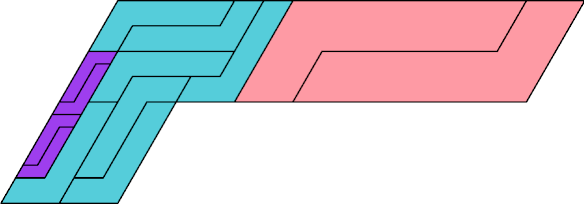 |
| 13 | |

| 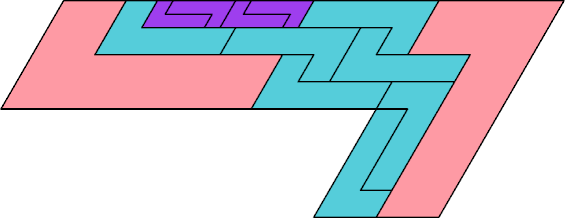 |
| 13 | |

|  |
| 31 | |

| 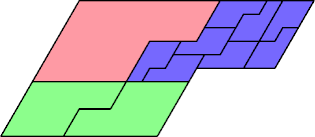 |
| 11 | |

| 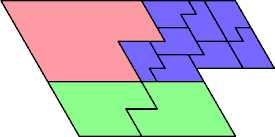 |
| 11 | |

| 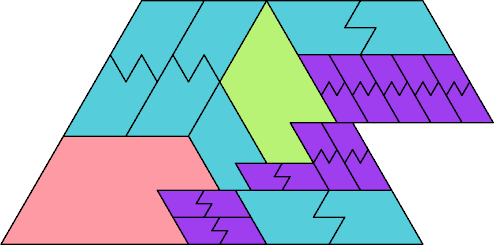 |
| 25 | |

| 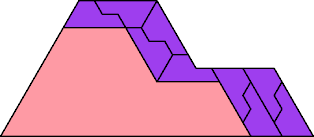 |
| 10 | |

| 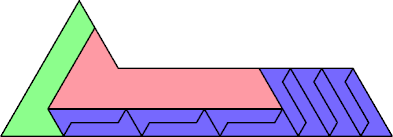 |
| 14 |

| 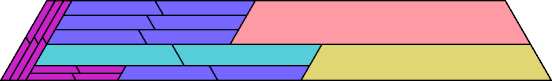 |
| 22 |

|  |
| 4 | |

|  |
| 12 | |

|  |
| 12 | |

|  |
| 21 | |

| 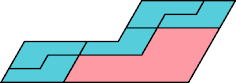 |
| 6 | |

| 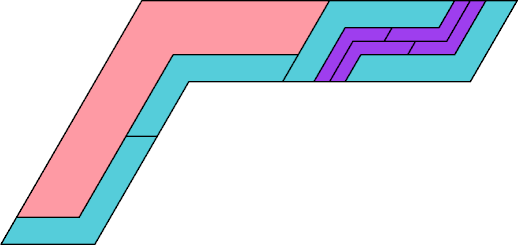 |
| 9 | |

|  |
| 18 | |

|  |
| 27 | |

|  |
| 18 | |

|  |
| 18 | |

|  |
| 18 | |

|  |
| 27 | |

|  |
| 6 | |

| 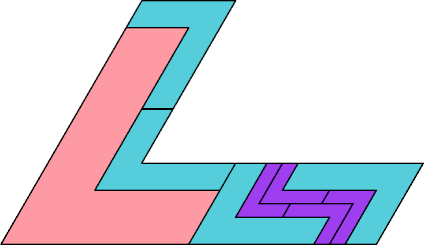 |
| 9 | |

|  |
| 15 | |

|  |
| 4 | |

|  |
| 8 | |

|  |
| 12 | |

|  |
| 27 | |

|  |
| 12 | |

|  |
| 12 | |

| 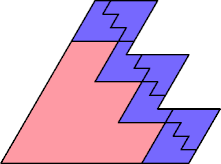 |
| 8 | |

|  |
| 18 | |

|  |
| 18 | |

|  |
| 11 | |

|  |
| 18 | |

|  |
| 27 | |

|  |
| 14 | |

|  |
| 27 | |

|  |
| 27 | |

|  |
| 27 | |

|  |
| 27 | |

| 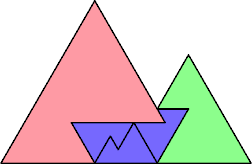
Karl Scherer |
| 5 |
Last revised 2025-04-02.