Tiling Right Trapezoidal Polyominoes with Three Pentominoes

  • Introduction
  • Nomenclature
  • Table
  • Solutions

    • Introduction

    A pentomino is a figure made of five squares joined edge to edge. There are 12 such figures, not distinguishing reflections and rotations. They were first enumerated and studied by Solomon Golomb.

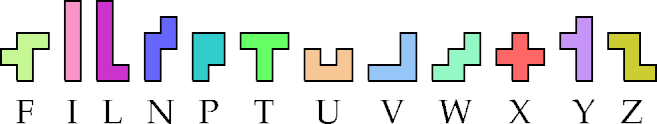
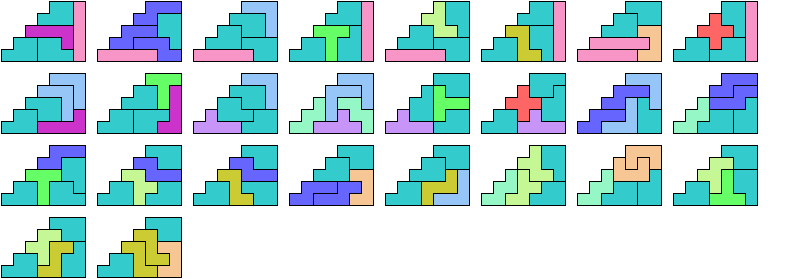
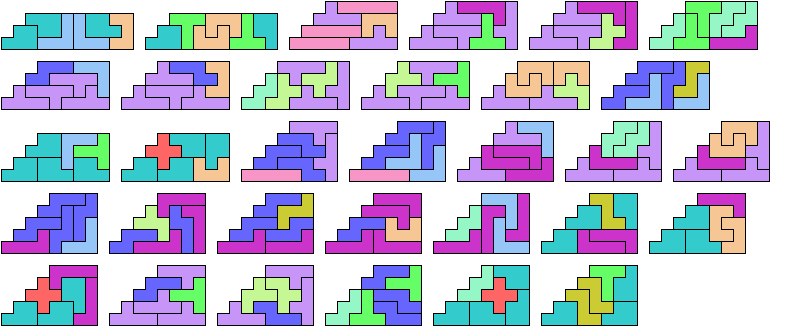
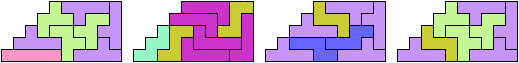
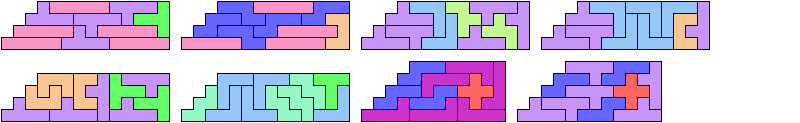
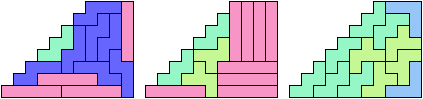
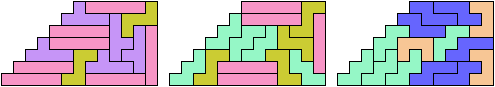
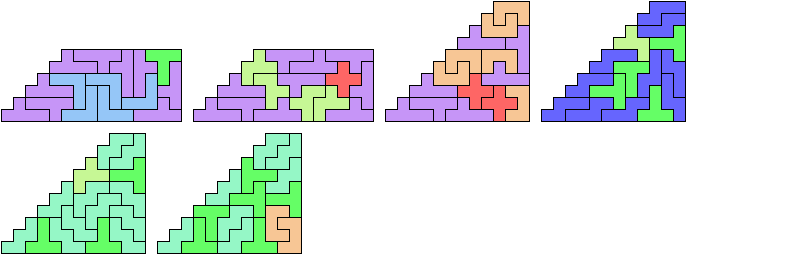
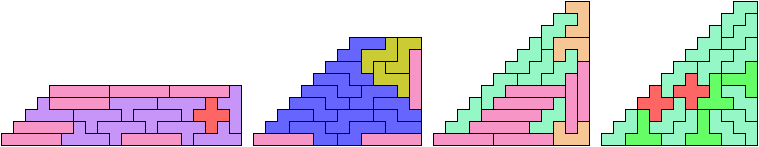
    Here I study the problem of tiling a polyomino shaped like a right trapezoid with copies of three pentominoes, using at least one of each. Such a polyomino has three straight sides, two of them parallel, and one zigzag side. For this problem, the polyomino may be triangular.

    If you find a smaller solution than one of mine or solve an unsolved case, please write!

    See also Tiling a Right Trapezoidal Polyomino with Two Pentominoes and L Shapes from Three Pentominoes.

    Nomenclature

    I use Solomon W. Golomb's original names for the pentominoes:

    Table

    This table shows the smallest total number of three pentominoes known to be able to tile a trapezoidal polyomino:

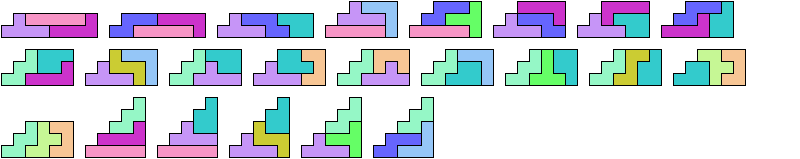
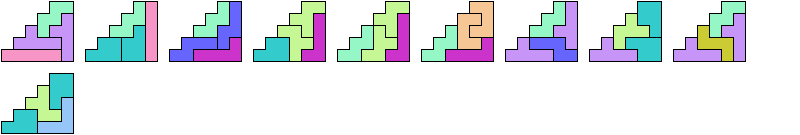
    FIL —FNV 9FUZ —INU 10IUY 6LPV 5LWZ 8NVW 3PVX 7TXZ —
    FIN 9FNW —FVW 12INV 6IUZ —LPW 3LXY 7NVX 20PVY 5TYZ —
    FIP 5FNX —FVX —INW 12IVW 11LPX 6LXZ —NVY 6PVZ 5UVW 27
    FIT —FNY 6FVY 10INX 27IVX —LPY 3LYZ 7NVZ 6PWX 6UVX —
    FIU —FNZ —FVZ —INY 6IVY 3LPZ 6NPT 5NWX —PWY 3UVY 10
    FIV —FPT 5FWX —INZ 18IVZ —LTU —NPU 5NWY 4PWZ 3UVZ —
    FIW 12FPU 3FWY 6IPT 5IWX 21LTV —NPV 5NWZ —PXY 5UWX —
    FIX —FPV 4FWZ —IPU 5IWY 4LTW 6NPW 5NXY 10PXZ 7UWY 3
    FIY 8FPW 5FXY 15IPV 5IWZ 14LTX —NPX 7NXZ —PYZ 3UWZ —
    FIZ —FPX 7FXZ —IPW 4IXY 18LTY 6NPY 3NYZ 8TUV —UXY 15
    FLN 6FPY 4FYZ 8IPX 5IXZ —LTZ —NPZ 5PTU 6TUW 15UXZ —
    FLP 4FPZ 5ILN 3IPY 3IYZ 14LUV —NTU 9PTV 6TUX —UYZ 9
    FLT —FTU —ILP 5IPZ 5LNP 3LUW 4NTV 9PTW 3TUY 10VWX 35
    FLU —FTV —ILT —ITU —LNT 7LUX —NTW 6PTX 7TUZ —VWY 5
    FLV —FTW 15ILU —ITV —LNU 6LUY 6NTX 33PTY 5TVW 10VWZ 11
    FLW 4FTX —ILV —ITW 7LNV 6LUZ —NTY 6PTZ 6TVX —VXY 27
    FLX —FTY 6ILW 3ITX —LNW 4LVW 6NTZ 20PUV 6TVY 15VXZ —
    FLY 6FTZ —ILX —ITY 10LNX 10LVX —NUV 9PUW 5TVZ —VYZ 3
    FLZ —FUV —ILY 3ITZ —LNY 3LVY 6NUW 14PUX 6TWX 18WXY 7
    FNP 5FUW 3ILZ —IUV —LNZ 6LVZ —NUX —PUY 3TWY 3WXZ —
    FNT 15FUX —INP 5IUW 18LPT 5LWX 7NUY 6PUZ 5TWZ 11WYZ 4
    FNU —FUY 6INT 3IUX —LPU 6LWY 6NUZ —PVW 3TXY —XYZ —

    Solutions

    So far as I know, these solutions have the fewest possible tiles. They are not necessarily uniquely minimal.

    3 Tiles

    4 Tiles

    5 Tiles

    6 Tiles

    7 Tiles

    8 Tiles

    9 Tiles

    10 Tiles

    11 Tiles

    12 Tiles

    14 Tiles

    15 Tiles

    18 Tiles

    20 Tiles

    21 Tiles

    27 Tiles

    33 Tiles

    35 Tiles

    Last revised 2024-01-01.

    Back to Polyomino and Polyking Tiling < Polyform Tiling < Polyform Curiosities
    Col. George Sicherman [ HOME | MAIL ]