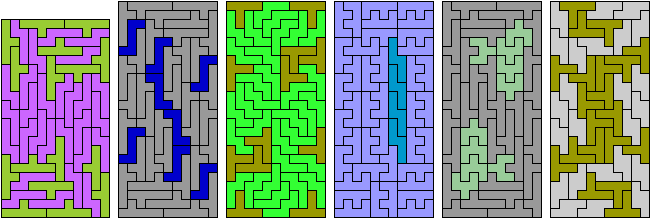
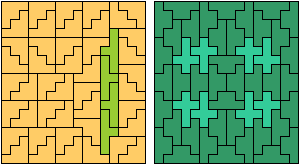
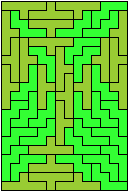
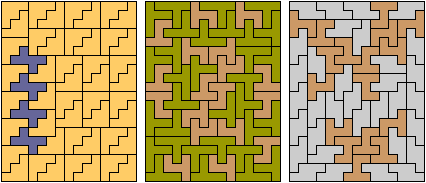
Mike Reid found the last two tilings. Patrick Hamlyn independently found the solutions with 48 or fewer tiles.
See also Scaled Hexomino Pair Rectangles.
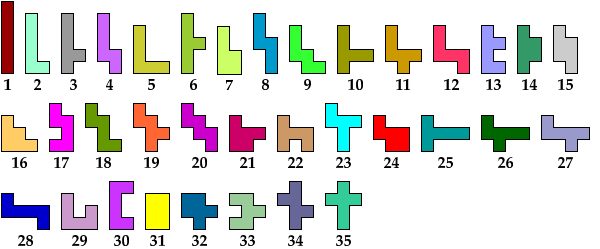
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 30 | 31 | 32 | 33 | 34 | 35 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | • | 3 | 12 | 14 | 4 | 14 | 3 | 14 | 39 | 14 | 39 | 14 | 5 | 5 | 14 | 5 | 36 | 14 | 32 | 39 | 14 | 39 | ? | 14 | 4 | 39 | 100 | 32 | 5 | 20 | 3 | 504 | 26 | ? | ? |
| 2 | 3 | • | 8 | 6 | 4 | 10 | 4 | 12 | 4 | 9 | 14 | 10 | 6 | 5 | 4 | 6 | 10 | 12 | 20 | 20 | 6 | 12 | 16 | 4 | 14 | 4 | 16 | 16 | 4 | 6 | 3 | 20 | 14 | 20 | 24 |
| 3 | 12 | 8 | • | 28 | 6 | 12 | 6 | 12 | 20 | 10 | 20 | 6 | 6 | 6 | 12 | 4 | 4 | 6 | 8 | 14 | 4 | 4 | 14 | 6 | 4 | 20 | 10 | 44 | 6 | 24 | 4 | 16 | 44 | 96 | 64 |
| 4 | 14 | 6 | 28 | • | 4 | 44 | 6 | × | × | 54 | × | × | 6 | 8 | × | 4 | 6 | × | × | × | 12 | 30 | × | × | 16 | × | × | × | 4 | 28 | 12 | × | ? | × | × |
| 5 | 4 | 4 | 6 | 4 | • | 42 | 4 | ? | 26 | 72 | ? | 4 | 4 | 8 | ? | 4 | 18 | 4 | 6 | ? | 4 | 10 | 4 | 4 | 12 | 6 | 12 | 3 | 4 | 20 | 2 | 6 | ? | ? | ? |
| 6 | 14 | 10 | 12 | 44 | 42 | • | 3 | ? | 49 | 150 | ? | 28 | 18 | 20 | 94 | 48 | 6 | 24 | 14 | ? | 4 | 102 | 8 | 14 | ? | 96 | ? | ? | 6 | ? | 8 | 164 | 96 | ? | ? |
| 7 | 3 | 4 | 6 | 6 | 4 | 3 | • | 3 | 8 | 4 | 10 | 4 | 4 | 6 | 12 | 4 | 14 | 4 | 6 | 24 | 4 | 7 | 5 | 4 | 10 | 8 | 6 | 5 | 4 | 5 | 3 | 6 | 24 | 8 | 12 |
| 8 | 14 | 12 | 12 | × | ? | ? | 3 | • | × | 400 | × | × | 44 | 30 | × | 40 | ? | × | × | × | 24 | ? | × | × | ? | × | × | × | 4 | ? | 5 | × | ? | × | × |
| 9 | 39 | 4 | 20 | × | 26 | 49 | 8 | × | • | 44 | × | × | 20 | 6 | × | 12 | ? | × | × | × | 6 | ? | × | × | ? | × | × | × | 28 | 66 | 12 | × | ? | × | × |
| 10 | 14 | 9 | 10 | 54 | 72 | 150 | 4 | 400 | 44 | • | ? | 56 | 14 | 4 | 44 | 28 | 152 | 6 | ? | ? | 16 | 50 | ? | 8 | ? | ? | 96 | ? | 6 | 136 | 4 | 80 | ? | ? | ? |
| 11 | 39 | 14 | 20 | × | ? | ? | 10 | × | × | ? | • | × | 4 | 42 | × | 28 | 100 | × | × | × | 18 | 14 | × | × | ? | × | × | × | 28 | ? | 12 | × | ? | × | × |
| 12 | 14 | 10 | 6 | × | 4 | 28 | 4 | × | × | 56 | × | • | 24 | 30 | × | 25 | ? | × | × | × | 16 | ? | × | × | ? | × | × | × | 25 | 16 | 6 | × | ? | × | × |
| 13 | 5 | 6 | 6 | 6 | 4 | 18 | 4 | 44 | 20 | 14 | 4 | 24 | • | 4 | 4 | 4 | 6 | 39 | 14 | 14 | 6 | 4 | 22 | 40 | 14 | 14 | 14 | 25 | 4 | 20 | 3 | 4 | 10 | 14 | 10 |
| 14 | 5 | 5 | 6 | 8 | 8 | 20 | 6 | 30 | 6 | 4 | 42 | 30 | 4 | • | 6 | 3 | 20 | 15 | 18 | 5 | 4 | 24 | 42 | 8 | 15 | 20 | 30 | 30 | 3 | 2 | 6 | 10 | 18 | 36 | 48 |
| 15 | 14 | 4 | 12 | × | ? | 94 | 12 | × | × | 44 | × | × | 4 | 6 | • | 4 | ? | × | × | × | 10 | 50 | × | × | 14 | × | × | × | 4 | ? | 12 | × | ? | × | × |
| 16 | 5 | 6 | 4 | 4 | 4 | 48 | 4 | 40 | 12 | 28 | 28 | 25 | 4 | 3 | 4 | • | 16 | 40 | 14 | 3 | 8 | 40 | 42 | 6 | 14 | 14 | 14 | 14 | 4 | 77 | 3 | 8 | 14 | 50 | 132 |
| 17 | 36 | 10 | 4 | 6 | 18 | 6 | 14 | ? | ? | 152 | 100 | ? | 6 | 20 | ? | 16 | • | 120 | 100 | ? | 4 | ? | ? | ? | ? | 64 | 168 | ? | 3 | 6 | 14 | 54 | 14 | ? | ? |
| 18 | 14 | 12 | 6 | × | 4 | 24 | 4 | × | × | 6 | × | × | 39 | 15 | × | 40 | 120 | • | × | × | 18 | ? | × | × | ? | × | × | × | 40 | 80 | 12 | × | ? | × | × |
| 19 | 32 | 20 | 8 | × | 6 | 14 | 6 | × | × | ? | × | × | 14 | 18 | × | 14 | 100 | × | • | × | 12 | ? | × | × | ? | × | × | × | 4 | ? | 14 | × | ? | × | × |
| 20 | 39 | 20 | 14 | × | ? | ? | 24 | × | × | ? | × | × | 14 | 5 | × | 3 | ? | × | × | • | 12 | ? | × | × | ? | × | × | × | 40 | ? | ? | × | ? | × | × |
| 21 | 14 | 6 | 4 | 12 | 4 | 4 | 4 | 24 | 6 | 16 | 18 | 16 | 6 | 4 | 10 | 8 | 4 | 18 | 12 | 12 | • | 10 | 30 | 10 | 28 | 22 | 24 | 24 | 2 | 16 | 10 | 18 | 10 | 28 | 36 |
| 22 | 39 | 12 | 4 | 30 | 10 | 102 | 7 | ? | ? | 50 | 14 | ? | 4 | 24 | 50 | 40 | ? | ? | ? | ? | 10 | • | ? | ? | ? | ? | ? | ? | 14 | 112 | 12 | 28 | ? | ? | ? |
| 23 | ? | 16 | 14 | × | 4 | 8 | 5 | × | × | ? | × | × | 22 | 42 | × | 42 | ? | × | × | × | 30 | ? | • | × | ? | × | × | × | 8 | 70 | 12 | × | ? | × | × |
| 24 | 14 | 4 | 6 | × | 4 | 14 | 4 | × | × | 8 | × | × | 40 | 8 | × | 6 | ? | × | × | × | 10 | ? | × | • | 40 | × | × | × | 30 | 4 | 10 | × | ? | × | × |
| 25 | 4 | 14 | 4 | 16 | 12 | ? | 10 | ? | ? | ? | ? | ? | 14 | 15 | 14 | 14 | ? | ? | ? | ? | 28 | ? | ? | 40 | • | ? | ? | ? | 14 | ? | 12 | ? | ? | ? | ? |
| 26 | 39 | 4 | 20 | × | 6 | 96 | 8 | × | × | ? | × | × | 14 | 20 | × | 14 | 64 | × | × | × | 22 | ? | × | × | ? | • | × | × | 20 | ? | 15 | × | ? | × | × |
| 27 | 100 | 16 | 10 | × | 12 | ? | 6 | × | × | 96 | × | × | 14 | 30 | × | 14 | 168 | × | × | × | 24 | ? | × | × | ? | × | • | × | 30 | ? | 18 | × | ? | × | × |
| 28 | 32 | 16 | 44 | × | 3 | ? | 5 | × | × | ? | × | × | 25 | 30 | × | 14 | ? | × | × | × | 24 | ? | × | × | ? | × | × | • | 40 | ? | 12 | × | ? | × | × |
| 29 | 5 | 4 | 6 | 4 | 4 | 6 | 4 | 4 | 28 | 6 | 28 | 25 | 4 | 3 | 4 | 4 | 3 | 40 | 4 | 40 | 2 | 14 | 8 | 30 | 14 | 20 | 30 | 40 | • | 3 | 3 | 96 | 56 | 3 | 56 |
| 30 | 20 | 6 | 24 | 28 | 20 | ? | 5 | ? | 66 | 136 | ? | 16 | 20 | 2 | ? | 77 | 6 | 80 | ? | ? | 16 | 112 | 70 | 4 | ? | ? | ? | ? | 3 | • | 16 | ? | ? | ? | ? |
| 31 | 3 | 3 | 4 | 12 | 2 | 8 | 3 | 5 | 12 | 4 | 12 | 6 | 3 | 6 | 12 | 3 | 14 | 12 | 14 | ? | 10 | 12 | 12 | 10 | 12 | 15 | 18 | 12 | 3 | 16 | • | 20 | ? | 60 | 24 |
| 32 | 504 | 20 | 16 | × | 6 | 164 | 6 | × | × | 80 | × | × | 4 | 10 | × | 8 | 54 | × | × | × | 18 | 28 | × | × | ? | × | × | × | 96 | ? | 20 | • | ? | × | × |
| 33 | 26 | 14 | 44 | ? | ? | 96 | 24 | ? | ? | ? | ? | ? | 10 | 18 | ? | 14 | 14 | ? | ? | ? | 10 | ? | ? | ? | ? | ? | ? | ? | 56 | ? | ? | ? | • | ? | ? |
| 34 | ? | 20 | 96 | × | ? | ? | 8 | × | × | ? | × | × | 14 | 36 | × | 50 | ? | × | × | × | 28 | ? | × | × | ? | × | × | × | 3 | ? | 60 | × | ? | • | × |
| 35 | ? | 24 | 64 | × | ? | ? | 12 | × | × | ? | × | × | 10 | 48 | × | 132 | ? | × | × | × | 36 | ? | × | × | ? | × | × | × | 56 | ? | 24 | × | ? | × | • |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | 15 | 16 | 17 | 18 | 19 | 20 | 21 | 22 | 23 | 24 | 25 | 26 | 27 | 28 | 29 | 30 | 31 | 32 | 33 | 34 | 35 |


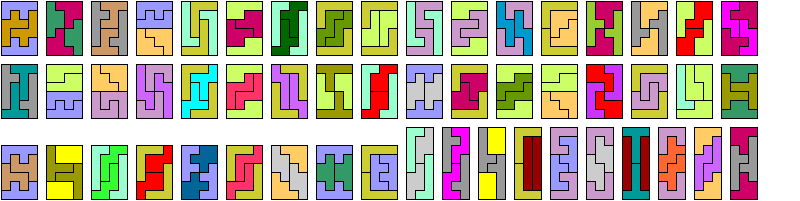
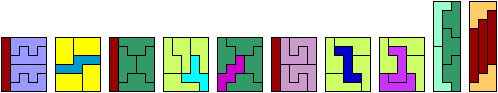
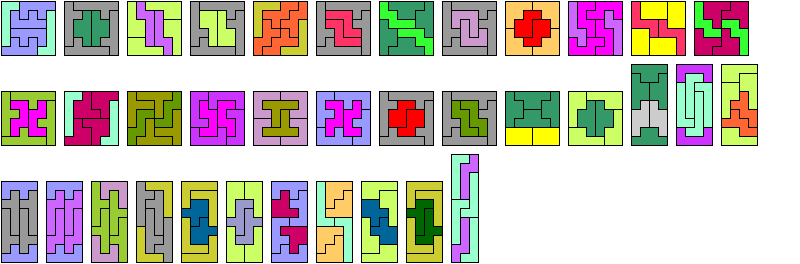












 Last revised 2022-11-18.
Last revised 2022-11-18.