Holeless Hexomino Compatibility

Introduction

A hexomino is a figure made of six squares joined edge to edge. There are 35 such figures, not distinguishing reflections and rotations. They were first enumerated and studied by Solomon Golomb.

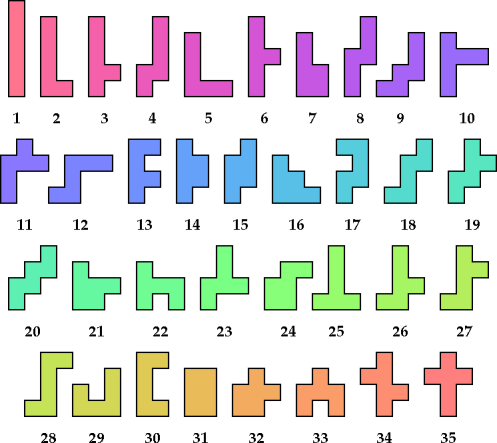
The compatibility problem is to find a figure that can be tiled with each of a set of polyforms. Polyomino compatibility has been widely studied since the early 1990s, and two well-known websites, Poly2ominoes by Jorge Mireles and Polypolyominoes by Giovanni Resta, present the results of their authors' systematic searches for compatibility figures. The sites include solutions by other researchers, especially Mike Reid. So far as I know, polyomino compatibility has not been treated in print since Golomb first raised the issue in 1981, except in a series of articles called Polyomino Number Theory, written by Andris Cibulis, Andy Liu, Bob Wainwright, Uldis Barbans, and Gilbert Lee from 2002 to 2005.

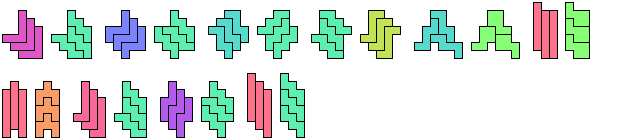
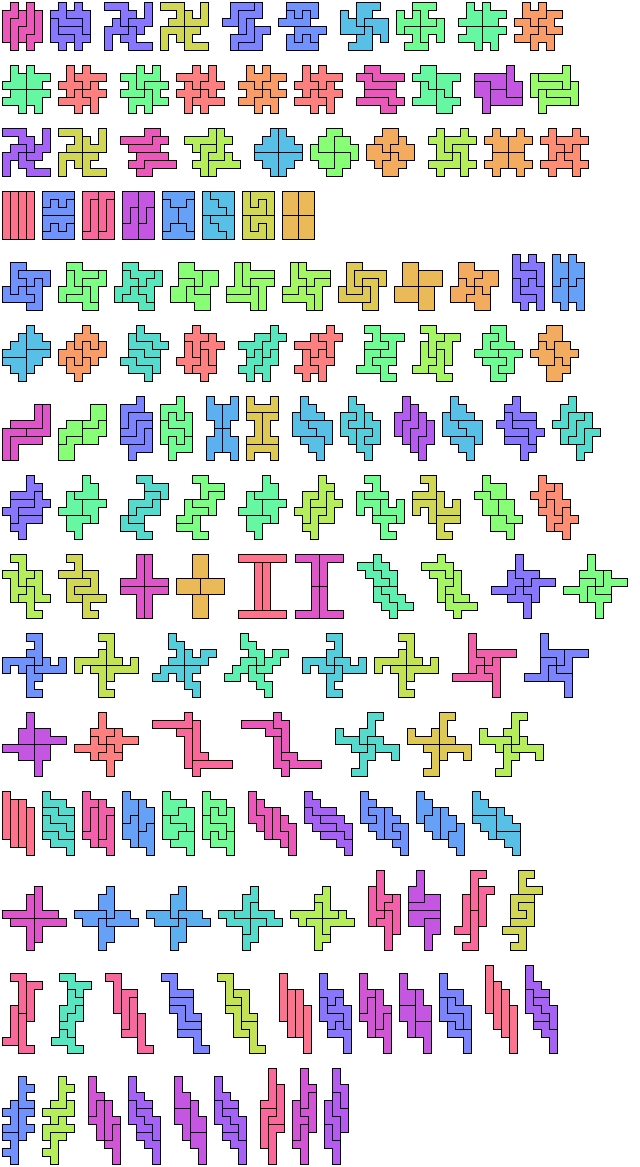
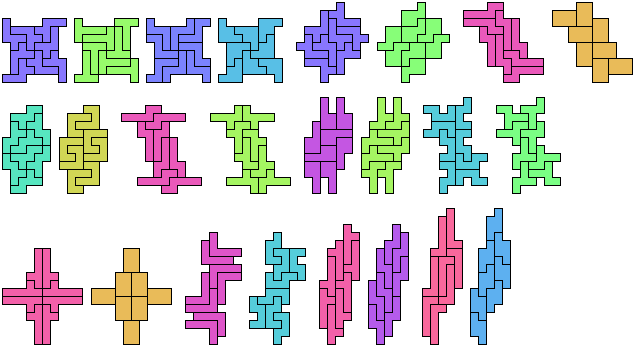
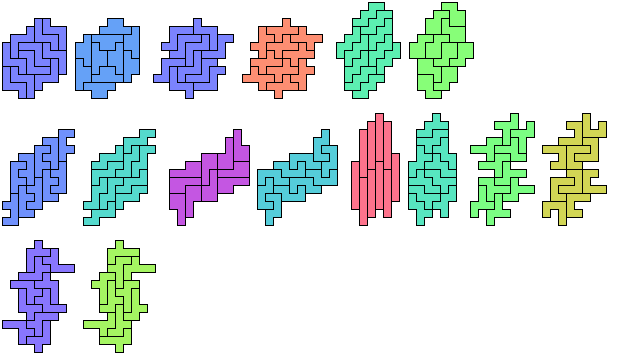
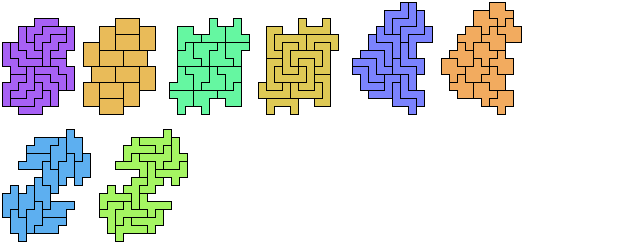
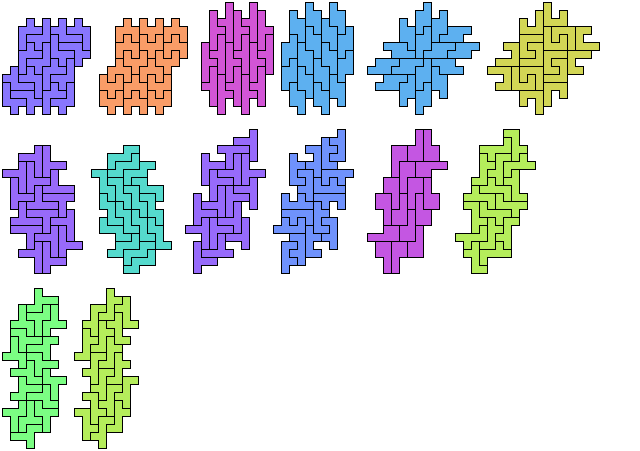
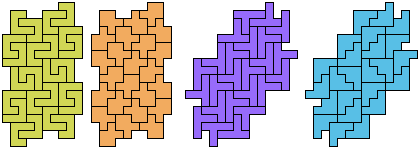
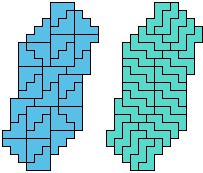
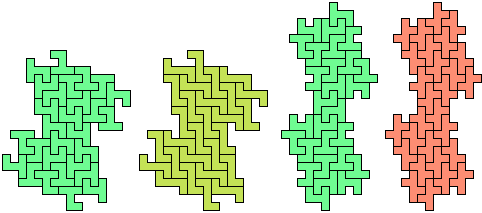
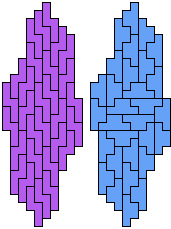
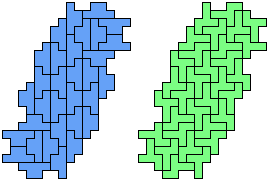
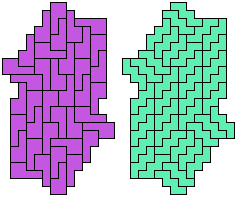
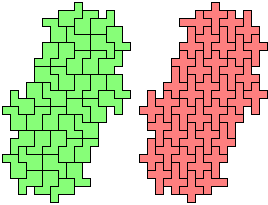
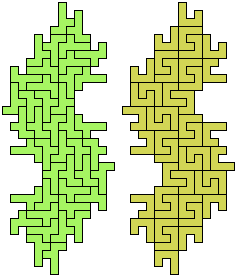
The websites and the articles show only minimal solutions with no restriction. Here I show minimal known hexomino compatibility figures without holes. If you find a smaller solution or solve an unsolved case, please let me know.

I am grateful to V. Pocins for improving one of the solutions on this page, and for suggesting improvements to the text.

For pentomino compatibility with or without holes, see Pentomino Compatibility. For hexomino compatibility allowing holes, see Resta's Hexominoes. For pentomino-hexomino compatibility without holes, see Holeless Pentomino-Hexomino Compatibility.

Numbers

I number the hexominoes from 1 to 35 as shown:

Table

This table shows the smallest number of tiles known to suffice to construct a holeless figure tilable by both hexominoes. A × means that there is almost surely no solution. (It is rarely possible to prove impossibility by exhaustion.) A ? means that no solution is known to me. Cells shaded in green require the same number of tiles when holes are allowed.

 1234567891011121314151617181920212223242526272829303132333435
1*22242224?444224?410344×3?6?×4?2×3××
22*2424242224248222432622222442218?××
322*222482?242222?42624?622222?82622
4242*2?6?4?42222222224222284?212862××
54222*182?22226422841232664224?2?4??××
6242?18*224?44??16×?212×2?22×22?2????22
7224622*24242246210223822224816?2622??4
8248??22*2?22?30242?232?????×?2??×××?
942242442*226442422222262?212?4?1466××
10?2??2?2?2*22162?24?166×2?26?212?62??×?2
114224244222*22428?42?42488102?4??21622
1244422422622*4108452832422852222214?10?
1342226?2?41624*22221022224244442224222
1424224?430424102*22?42224322?64?42422××
152822216622?2822*2222622366?144?164??6×?
1642222×2442484222*4262224?4282?2?244××
17?2?28?1022??52?24*2242286?2242??????
184242422?216421042262*232243?6242422624
19104221212222628222222*2222244248242224
2033623×3832×?32262432*218?10×4123×?×22××
2142242222224222222222*222224221422424
2246426?2?2?242424222182*22242284??42284
23×2?2622?624243236?842?22*24416?10244?122
243262422?2682226463210222*422464428440
25?2222×4???884??2??4×2244*28?12444××?
266228228?2210546148264424422*22424422?2
27?2244216×1212224442222124216282*244?4?2?
28×42????????24???4443228?4?22*2×?2×××
2944222222464224162228×24106124242*2224×××
30?2?12??6??2?2224??42?14?24444×2*44×××
3122884?2?14??224?2?24×2?4444??24*4×××
32×1826??2×6?21442?4?222244242422444*242
333?62???×6×16?2264?62242?8×2?××××2*?4
34××2××2?××?2102×××?22×228124×?2××××4?*2
35××2××24?×22?2×?×?44×44240?2?××××242*
 1234567891011121314151617181920212223242526272829303132333435

Solutions

So far as I know, these solutions are minimal. They are not necessarily uniquely minimal.

  • 2 Tiles
  • 3 Tiles
  • 4 Tiles
  • 5 Tiles
  • 6 Tiles
  • 8 Tiles
  • 10 Tiles
  • 12 Tiles
  • 14 Tiles
  • 16 Tiles
  • 18 Tiles
  • 24 Tiles
  • 26 Tiles
  • 28 Tiles
  • 30 Tiles
  • 32 Tiles
  • 36 Tiles
  • 38 Tiles
  • 40 Tiles
  • 42 Tiles

    • 2 Tiles

    3 Tiles

    4 Tiles

    5 Tiles

    6 Tiles

    8 Tiles

    10 Tiles

    12 Tiles

    14 Tiles

    16 Tiles

    18 Tiles

    24 Tiles

    26 Tiles

    28 Tiles

    30 Tiles

    32 Tiles

    36 Tiles

    38 Tiles

    40 Tiles

    42 Tiles

    Last revised 2020-06-18.

    Back to Pairwise Compatibility < Polyform Compatibility < Polyform Curiosities
    Col. George Sicherman [ HOME | MAIL ]