 |
 |
 |
 |
 |
There are 880 basic magic squares of order-4. The complete set was compiled by Bernard
Frénicle de Bessy before 1675. [1][2]
This list has been recalculated and verified by many people since that time (myself
included).
These 880 magic squares were classified into 12 groups by H. E. Dudeney and first published in The Queen, Jan. 15, 1910. The classification diagrams appeared later in Amusements in Mathematics, 1917 published by Thomas Nelson & Sons, Ltd.
Both the list of magic squares and the group classification has been more recently published in William H. Benson and Oswald Jacoby, New Recreations with Magic Squares, Dover Publ., 1976, 0-486-23236-0.
[1] Frénicle de Bessy, Des Quarrez ou Tables Magiques,
including: Table generale des quarrez de quatre. Mem. de l’Acad. Roy. des
Sc. 5 (1666-1699) (1729) 209-354. (Frénicle died in 1675).
[2] B.
Frénicle de Bessy, et al., Divers ouvrages de mathematique et de physique
(1693).
Ollerenshaw & Bondi cite a 1731 edition from The Hague??) (= Divers Ouvrages de
Mathématique et de Physique par Messieurs de l’Académie des Sciences; ed. P. de
la Hire; and Paris, 1693, pp. 423-507, ??NYS. (Rara, 632). Recueil
de divers Ouvrages de Mathematique de Mr. Frenicle.
B. Frénicle de Bessy, Traité des triangles rectangles en nombres, dans lequel plusieurs
belles proprietés de ces triangles sont demontrées
par de nouveaux principes (1676) did NOT contain any magic squares. (Paul
Pasles email Jan. 14, 2003).
![]()
The 12 groups
The 12 groups are classified by the patterns formed by the 8 complement pairs.
A complement pair is two numbers that together sum to n2 + 1. For
order-4 that number is 17.
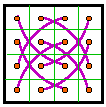 Group I |
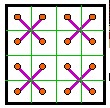 Group II |
 Group III |
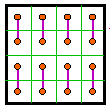 Group IV |
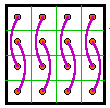 Group V |
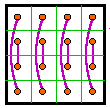 Group VI |
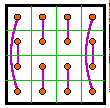 Group VII |
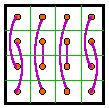 Group VIII |
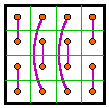 Group IX |
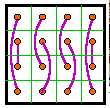 Group X |
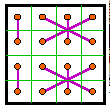 Group XI |
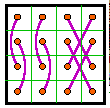 Group XII |
Groups III and VI are self-similar. That is, when each number is complemented, the same magic square is generated (only in a different orientation).
The twelve groups themselves may be grouped into four sets in which the groups in each set are strongly related. They are:
Set |
Group |
Number of |
Features |
Symmetry |
| 1 | I | 48 | pandiagonal | Set 1 - |
| 1 | II | 48 | semi-pandiagonal, bent-diagonal | symmetrical around the four quadrant centers |
| 1 | III | 48 | semi-pandiagonal, associative | symmetrical around the center point |
| 2 | IV | 96 | semi-pandiagonal | Set 2 |
| 2 | V | 96 | semi-pandiagonal | . |
| 2 | VI-P | 96 | semi-pandiagonal | symmetrical across the center line |
| 3 | VI-S | 208 | simple | Set 3 - symmetrical across the center line |
| 3 | VII | 56 | simple | . |
| 3 | VIII | 56 | simple | |
| 3 | IX | 56 | simple | |
| 3 | X | 56 | simple | |
| 4 | XI | 8 | simple | Set 4 |
| 4 | XII | 8 | simple | ......... |
The members of each set have many features in common that become evident
when working with transitions.
Also sets 1 and 2 are closely related as evidenced by the fact that 30 of the 48
transformations listed on the Transformations Summary
page work for all six groups of these two sets.
Sets 2 and 3 have 2 orientations of the complementary pair pattern. 0° and 90°.
Set 4 each group has 3 orientations. Group XI
has 0°, 180° and 270°. Group XII has 0°, 90° and 180° .
On this page I have posted all order-4 magic squares of the five smallest groups, which also happen to be the most interesting.
The four following pages contain the entire
list of 880 solutions. They appear one solution per line, in index order. Each line
includes the Dudeney group with degree of rotation required and the complement pair number
and partner solution,
Each page is quite large, so be patient while it loads.
Two files are available
for download:
One is sorted in index order, the other is also in index order but sorted into the 12
different groups.
Some Order-4 Features
The 4 corners of all order-4 squares sum to the constant S. The four central cells also sum to S.
All order-4 squares contain 2 even and 2 odd corners.
The four corner 2 x 2 arrays of all Groups I to Group VI-P squares (432 squares) sum to S. i.e. all these are gnomon-magic squares.
![]()
Group I ...The pandiagonals |
The 48 pandiagonal magic squares of order-4. |
Group II ...The bent diagonals |
The 48 bent diagonal semi-pandiagonal magic squares of order-4. |
Group III …The symmetricals |
The 48 associated semi-pandiagonal magic squares of order-4. |
Groups XI and XII …the odd balls |
The 8 magic squares for each of these groups with limited symmetry. |
List of Solutions - # 1 to 200 |
200 of the 880 basic order-4 magic squares in index order |
List of Solutions - # 201 to 400 |
200 of the 880 basic order-4 magic squares in index order |
List of Solutions - # 401 to 600 |
200 of the 880 basic order-4 magic squares in index order |
List of Solutions - # 601 to 880 |
280 of the 880 basic order-4 magic squares in index order |
Intro to Order-4 Transforms. |
Back to the introduction page to 48 transformations. (Also up arrow above and at end). |
![]()
A note regarding Groups I, II and III.
These are the most feature rich magic squares of order-4. In fact, pandiagonal magic
squares are also known as perfect.
All the magic squares of Groups I, II and III have the feature that the corner cells of
many 2x2 (i.e. all the cells), and all 3x3 and 4x4 squares sum to 34 (the magic constant).
Refer to the note following the listing for each group to realize other closely related
features!
These are the 48 pandiagonal magic squares of order-4. They are scattered throughout the 880 magic squares of this order. The number above each square is the position in the indexed list. The letters A, B, C indicate which of 3 sets of 16 that square belongs to.
 |
Following are the 48 pandiagonal magic squares of order-4. The image on the left is the Dudeney pattern for this group, showing the complement pairs. These 48 magic squares may be divided into 3 sets of 16 (A, B, C). Any square in a set may be transformed to any other square in the same set by moving rows and/or columns from one side of the square to the other. However, in most cases the resulting square will be rotated and/or reflected from the basic magic square shown here. Set B is shown that way on my Transformations page. |
#102 A #104 A #107 B #109 B #116 C #117 C 1 8 10 15 1 8 10 15 1 8 11 14 1 8 11 14 1 8 13 12 1 8 13 12 12 13 3 6 14 11 5 4 12 13 2 7 15 10 5 4 14 11 2 7 15 10 3 6 7 2 16 9 7 2 16 9 6 3 16 9 6 3 16 9 4 5 16 9 4 5 16 9 14 11 5 4 12 13 3 6 15 10 5 4 12 13 2 7 15 10 3 6 14 11 2 7 #171 B #174 A #177 C #178 C #201 A #204 B 1 12 6 15 1 12 7 14 1 12 13 8 1 12 13 8 1 14 7 12 1 14 11 8 14 7 9 4 15 6 9 4 14 7 2 11 15 6 3 10 15 4 9 6 15 4 5 10 11 2 16 5 10 3 16 5 4 9 16 5 4 9 16 5 10 5 16 3 6 9 16 3 8 13 3 10 8 13 2 11 15 6 3 10 14 7 2 11 8 11 2 13 12 7 2 13 #279 A #281 A #292 B #294 B #304 C #305 C 2 7 9 16 2 7 9 16 2 7 12 13 2 7 12 13 2 7 14 11 2 7 14 11 11 14 4 5 13 12 6 3 11 14 1 8 16 9 6 3 13 12 1 8 16 9 4 5 8 1 15 10 8 1 15 10 5 4 15 10 5 4 15 10 3 6 15 10 3 6 15 10 13 12 6 3 11 14 4 5 16 9 6 3 11 14 1 8 16 9 4 5 13 12 1 8 #355 B #365 A #372 C #375 C #393 A #396 B 2 11 5 16 2 11 8 13 2 11 14 7 2 11 14 7 2 13 8 11 2 13 12 7 13 8 10 3 16 5 10 3 13 8 1 12 16 5 4 9 16 3 10 5 16 3 6 9 12 1 15 6 9 4 15 6 3 10 15 6 3 10 15 6 9 6 15 4 5 10 15 4 7 14 4 9 7 14 1 12 16 5 4 9 13 8 1 12 7 12 1 14 11 8 1 14 #469 B #473 A #483 C #485 C #530 A #532 B 3 6 9 16 3 6 12 13 3 6 15 10 3 6 15 10 3 10 5 16 3 10 8 13 13 12 7 2 16 9 7 2 13 12 1 8 16 9 4 5 13 8 11 2 16 5 11 2 8 1 14 11 5 4 14 11 2 7 14 11 2 7 14 11 12 1 14 7 9 4 14 7 10 15 4 5 10 15 1 8 16 9 4 5 13 12 1 8 6 15 4 9 6 15 1 12 #536 C #537 C #560 B #565 A #621 B #623 A 3 10 15 6 3 10 15 6 3 13 8 10 3 13 12 6 4 5 10 15 4 5 11 14 13 8 1 12 16 5 4 9 16 2 11 5 16 2 7 9 14 11 8 1 15 10 8 1 2 11 14 7 2 11 14 7 9 7 14 4 5 11 14 4 7 2 13 12 6 3 13 12 16 5 4 9 13 8 1 12 6 12 1 15 10 8 1 15 9 16 3 6 9 16 2 7 #646 C #647 C #690 A #691 B #702 C #704 C 4 5 16 9 4 5 16 9 4 9 6 15 4 9 7 14 4 9 16 5 4 9 16 5 14 11 2 7 15 10 3 6 14 7 12 1 15 6 12 1 14 7 2 11 15 6 3 10 1 8 13 12 1 8 13 12 11 2 13 8 10 3 13 8 1 12 13 8 1 12 13 8 15 10 3 6 14 11 2 7 5 16 3 10 5 16 2 11 15 6 3 10 14 7 2 11 #744 B #748 A #785 A #788 B #828 A #839 B 4 14 7 9 4 14 11 5 5 4 14 11 5 4 15 10 6 3 13 12 6 3 16 9 15 1 12 6 15 1 8 10 16 9 7 2 16 9 6 3 15 10 8 1 15 10 5 4 10 8 13 3 6 12 13 3 3 6 12 13 2 7 12 13 4 5 11 14 1 8 11 14 5 11 2 16 9 7 2 16 10 15 1 8 11 14 1 8 9 16 2 7 12 13 2 7
Of the 48 Group I magic squares, there are 12 pairs where lines 1 and 3 are identical. In each case, lines 2 and 4 are also identical but interchanged. Refer to the notes at end of group II and group III listings to see the close relationship between the 3 groups.
Group II ...The bent diagonals
 |
Following are the 48 magic squares of order-4, Group II. The magic squares in this group are all semi-pandiagonal and have the additional characteristic of magic bent diagonals. For # 21 (below) these are 1, 16, 2,15; 15, 2 11, 6, 6, 11, 5, 12; 12, 5, 16, 1 and their reverses such as 13, 4, 14, 3. The image on the left is the Dudeney pattern for this group, showing the complement pairs. |
#21 #22 #27 #28 #56 #57 1 4 14 15 1 4 14 15 1 4 15 14 1 4 15 14 1 6 12 15 1 6 12 15 13 16 2 3 13 16 2 3 13 16 3 2 13 16 3 2 11 16 2 5 11 16 2 5 8 5 11 10 12 9 7 6 8 5 10 11 12 9 6 7 8 3 13 10 14 9 7 4 12 9 7 6 8 5 11 10 12 9 6 7 8 5 10 11 14 9 7 4 8 3 13 10 #62 #63 #82 #83 #89 #90 1 6 15 12 1 6 15 12 1 7 12 14 1 7 12 14 1 7 14 12 1 7 14 12 11 16 5 2 11 16 5 2 10 16 3 5 10 16 3 5 10 16 5 3 10 16 5 3 8 3 10 13 14 9 4 7 8 2 13 11 15 9 6 4 8 2 11 13 15 9 4 6 14 9 4 7 8 3 10 13 15 9 6 4 8 2 13 11 15 9 4 6 8 2 11 13 #213 #214 #233 #234 #246 #247 2 3 13 16 2 3 13 16 2 3 16 13 2 3 16 13 2 5 11 16 2 5 11 16 14 15 1 4 14 15 1 4 14 15 4 1 14 15 4 1 12 15 1 6 12 15 1 6 7 6 12 9 11 10 8 5 7 6 9 12 11 10 5 8 7 4 14 9 13 10 8 3 11 10 8 5 7 6 12 9 11 10 5 8 7 6 9 12 13 10 8 3 7 4 14 9 #269 #270 #316 #317 #323 #324 2 5 16 11 2 5 16 11 2 8 11 13 2 8 11 13 2 8 13 11 2 8 13 11 12 15 6 1 12 15 6 1 9 15 4 6 9 15 4 6 9 15 6 4 9 15 6 4 7 4 9 14 13 10 3 8 7 1 14 12 16 10 5 3 7 1 12 14 16 10 3 5 13 10 3 8 7 4 9 14 16 10 5 3 7 1 14 12 16 10 3 5 7 1 12 14 #421 #422 #445 #446 #450 #464 3 2 13 16 3 2 13 16 3 2 16 13 3 2 16 13 3 5 10 16 3 5 16 10 15 14 1 4 15 14 1 4 15 14 4 1 15 14 4 1 12 14 1 7 12 14 7 1 6 7 12 9 10 11 8 5 6 7 9 12 10 11 5 8 13 11 8 2 6 4 9 15 10 11 8 5 6 7 12 9 10 11 5 8 6 7 9 12 6 4 15 9 13 11 2 8 #465 #503 #505 #506 #583 #584 3 5 16 10 3 8 10 13 3 8 13 10 3 8 13 10 4 1 14 15 4 1 14 15 12 14 7 1 9 14 4 7 9 14 7 4 9 14 7 4 16 13 2 3 16 13 2 3 13 11 2 8 16 11 5 2 6 1 12 15 16 11 2 5 5 8 11 10 9 12 7 6 6 4 9 15 6 1 15 12 16 11 2 5 6 1 12 15 9 12 7 6 5 8 11 10 #591 #592 #648 #661 #662 #668 4 1 15 14 4 1 15 14 4 6 9 15 4 6 15 9 4 6 15 9 4 7 9 14 16 13 3 2 16 13 3 2 11 13 2 8 11 13 8 2 11 13 8 2 10 13 3 8 5 8 10 11 9 12 6 7 14 12 7 1 5 3 10 16 14 12 1 7 15 12 6 1 9 12 6 7 5 8 10 11 5 3 16 10 14 12 1 7 5 3 10 16 5 2 16 11 #678 #679 #768 #779 #818 #844 4 7 14 9 4 7 14 9 5 2 16 11 5 3 16 10 6 1 15 12 6 4 15 9 10 13 8 3 10 13 8 3 15 12 6 1 14 12 7 1 16 11 5 2 13 11 8 2 5 2 11 16 15 12 1 6 4 7 9 14 4 6 9 15 3 8 10 13 3 5 10 16 15 12 1 6 5 2 11 16 10 13 3 8 11 13 2 8 9 14 4 7 12 14 1 7
Of the 48 Group II magic squares, there are 20 pairs where the first two lines are identical. In each case, lines 3 and 4 are also identical but interchanged.
Group III …The 48 symmetricals
 |
Following are the 48 associated
magic squares of order-4. These are also semi-pandiagonal. The image on the left is the Dudeney pattern for this group, showing the complement pairs. |
#112 #113 #120 #122 #124 #126 1 8 12 13 1 8 12 13 1 8 14 11 1 8 14 11 1 8 15 10 1 8 15 10 14 11 7 2 15 10 6 3 12 13 7 2 15 10 4 5 12 13 6 3 14 11 4 5 15 10 6 3 14 11 7 2 15 10 4 5 12 13 7 2 14 11 4 5 12 13 6 3 4 5 9 16 4 5 9 16 6 3 9 16 6 3 9 16 7 2 9 16 7 2 9 16 #175 #176 #183 #185 #203 #206 1 12 8 13 1 12 8 13 1 12 14 7 1 12 15 6 1 14 8 11 1 14 12 7 14 7 11 2 15 6 10 3 15 6 4 9 14 7 4 9 15 4 10 5 15 4 6 9 15 6 10 3 14 7 11 2 8 13 11 2 8 13 10 3 12 7 13 2 8 11 13 2 4 9 5 16 4 9 5 16 10 3 5 16 11 2 5 16 6 9 3 16 10 5 3 16 #289 #290 #297 #299 #306 #308 2 7 11 14 2 7 11 14 2 7 13 12 2 7 13 12 2 7 16 9 2 7 16 9 13 12 8 1 16 9 5 4 11 14 8 1 16 9 3 6 11 14 5 4 13 12 3 6 16 9 5 4 13 12 8 1 16 9 3 6 11 14 8 1 13 12 3 6 11 14 5 4 3 6 10 15 3 6 10 15 5 4 10 15 5 4 10 15 8 1 10 15 8 1 10 15 #360 #361 #368 #377 #392 #395 2 11 7 14 2 11 7 14 2 11 13 8 2 11 16 5 2 13 7 12 2 13 11 8 13 8 12 1 16 5 9 4 16 5 3 10 13 8 3 10 16 3 9 6 16 3 5 10 16 5 9 4 13 8 12 1 7 14 12 1 7 14 9 4 11 8 14 1 7 12 14 1 3 10 6 15 3 10 6 15 9 4 6 15 12 1 6 15 5 10 4 15 9 6 4 15 #476 #478 #487 #489 #535 #539 3 6 13 12 3 6 13 12 3 6 16 9 3 6 16 9 3 10 13 8 3 10 16 5 10 15 8 1 16 9 2 7 10 15 5 4 13 12 2 7 16 5 2 11 13 8 2 11 16 9 2 7 10 15 8 1 13 12 2 7 10 15 5 4 6 15 12 1 6 15 9 4 5 4 11 14 5 4 11 14 8 1 11 14 8 1 11 14 9 4 7 14 12 1 7 14 #558 #562 #628 #632 #635 #637 3 13 6 12 3 13 10 8 4 5 14 11 4 5 14 11 4 5 15 10 4 5 15 10 16 2 9 7 16 2 5 11 9 16 7 2 15 10 1 8 9 16 6 3 14 11 1 8 10 8 15 1 6 12 15 1 15 10 1 8 9 16 7 2 14 11 1 8 9 16 6 3 5 11 4 14 9 7 4 14 6 3 12 13 6 3 12 13 7 2 12 13 7 2 12 13 #695 #698 #741 #746 #789 #790 4 9 14 7 4 9 15 6 4 14 5 11 4 14 9 7 5 4 16 9 5 4 16 9 15 6 1 12 16 5 3 10 15 1 10 8 15 1 6 12 10 15 3 6 11 14 2 7 5 16 11 2 1 12 14 7 9 7 16 2 5 11 16 2 11 14 2 7 10 15 3 6 10 3 8 13 13 8 2 11 6 12 3 13 10 8 3 13 8 1 13 12 8 1 13 12 #803 #808 #834 #835 #850 #860 5 10 11 8 5 11 10 8 6 3 15 10 6 3 15 10 6 9 12 7 6 12 9 7 16 3 2 13 16 2 3 13 9 16 4 5 12 13 1 8 15 4 1 14 15 1 4 14 4 15 14 1 4 14 15 1 12 13 1 8 9 16 4 5 3 16 13 2 3 13 16 2 9 6 7 12 9 7 6 12 7 2 14 11 7 2 14 11 10 5 8 11 10 8 5 11
Of the 48 Group III magic squares, there are 13 pairs where lines 1 and
4 are identical. In each case, lines 2 and 3 are also identical but interchanged.
Pair 850/860 and 7 other pairs have columns 1 and 4 the same, columns 2 and 3
interchanged. There are only 6 of the 48 magic squares that do not belong to one or the
other of these two sets.
![]()
These two groups are the only ones not symmetrical around the horizontal and vertical center lines of the square. Consequently transformations to or from other groups will produce different results depending on the orientation of the particular magic square in these two groups.
Examining the main diagonals of the 8 group XI and eight group XII magic squares reveal
the similarity between these two groups. There are a total of nine sets of four numbers
that comprise the 32 main diagonals of these 16 magic squares. The 4 numbers in each set
may appear in different orders.
For group XII, the first 8 appear once in the first 4 magic squares and once in the second
4 magic squares.
Group XI is not nearly as ordered as group XII, as shown by the table.
| # | Set of 4 numbers | Group XI |
Group XII – 1st 4 |
Group XII – 2nd 4 |
| 1 | 1, 5, 13, 15 | X, X, X |
X |
X |
| 2 | 1, 9, 10, 14 | X |
X |
X |
| 3 | 2, 4, 12, 16 | X, X |
X |
X |
| 4 | 2, 9, 10, 13 | X, X |
X |
X |
| 5 | 3, 7, 8, 16 | X, X |
X |
X |
| 6 | 4, 7, 8, 15 | X, X |
X |
X |
| 7 | 4, 8, 10, 12 | X, X |
X |
X |
| 8 | 5, 7, 9, 13 | X |
X |
X |
| 9 | 6, 7, 9, 12 | X |
-- |
-- |
So can we say group XI is the most oddball oddball?
Group XI#181 #202 #364 #374 #484 #643 1 12 13 8 1 14 7 12 2 11 8 13 2 11 14 7 3 6 15 10 4 5 16 9 16 9 4 5 16 5 10 3 15 4 9 6 15 10 3 6 14 7 2 11 13 8 1 12 2 7 14 11 9 4 15 6 10 5 16 3 1 8 13 12 4 9 16 5 3 10 15 6 15 6 3 10 8 11 2 13 7 14 1 12 16 5 4 9 13 12 1 8 14 11 2 7
#689 #724 4 9 6 15 4 11 14 5 11 8 13 2 15 2 7 10 14 1 12 7 6 13 12 3 5 16 3 10 9 8 1 16 |
 |
Group 11 has Complementary Pair
(Dudeney) patterns with 3 orientations. No rotation: 181, 202, 364, 374, 484, 643 (the first 6). Rotated 180°: 689. Rotated 270°: 724. |
![]()
Group XII
#3 #88 #209 #319 #449 #613 1 2 16 15 1 7 14 12 2 1 15 16 2 8 11 13 3 4 14 13 4 3 13 14 13 14 4 3 9 15 4 6 14 13 3 4 10 16 5 3 15 16 2 1 16 15 1 2 12 7 9 6 16 10 5 3 11 8 10 5 15 9 4 6 6 9 7 12 5 10 8 11 8 11 5 10 8 2 11 13 7 12 6 9 7 1 14 12 10 5 11 8 9 6 12 7
#650 #666 4 6 9 15 4 6 15 9 14 12 1 7 14 12 7 1 11 13 8 2 11 13 2 8 5 3 16 10 5 3 10 16 |
 |
This group has magic squares with
three CP pattern orientations. No rotation: 88, 319. Rotated 90°: 3, 209, 449, 613. Rotated 180°: 650, 666. |
![]()
Please send me Feedback about my Web
site!
![]()
![]()
![]()

Last updated
July 14, 2008
Harvey Heinz harveyheinz@shaw.ca
Copyright © 2000 by Harvey D. Heinz