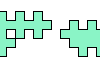Polyform Catalogues

    It was a strange collection, … but so much larger and so much more varied that I think I never had more pleasure than in sorting them.
—Robert Louis Stevenson, Treasure Island

  • Plane Figures With Grids
  • Plane Figures Without Grids
  • Solid Figures

    • Plane Figures With Grids

    Catalogue of Unitary Polyominoes. A unitary polyomino is one whose edges all have length 1.
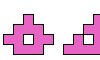    Catalogue of Almost Unitary Polyominoes. An almost unitary polyomino is one whose edges all but one have length 1.
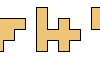    Catalogue of Almost Almost Unitary Polyominoes. An almost almost unitary polyomino is one whose edges all but two have length 1.
    Catalogue of Unitary Polyiamonds. A unitary polyiamond is one whose edges all have length 1.
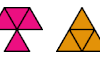    Catalogue of Polymings. A polyming is a generalization of a polyiamond in which cells may be joined at corners as well as at edges.
    Database of Convex Polyaboloes. A tarball of all convex polyaboloes with up to 800 cells.
    Catalogue of Blunt Polytans. A blunt polytan is one with no 45° corners.
    Catalogue of Tetrakis Polyaboloes. A tetrakis polyabolo is one that conforms to the Tetrakis Grid.
    Catalogue of Polyfetts. A polyfett is a generalized polyabolo whose cells may be joined at edges or at vertices.
    Catalogue of Convex Polydrafters.
    Catalogue of Didrifters.
    Catalogue of Polykagomes. A polykagome is a polyform defined on the trihexagonal grid.
    Catalogue of Polybirds. A polybird is a polyform defined on the rhombitrihexagonal grid.
    Catalogue of Polyhops. Thomas Atkinson's hopscotch-style polyominoes.
    Catalogue of Polyjogs. Polyforms formed of squares joined by half edges.
    Catalogue of Polynars. László Molnár's shapes formed of squares joined by edges and half edges.
    Catalogue of Polyhings. Polyforms formed of regular hexagons joined edge to edge or at vertices in parallel.

    Plane Figures Without Grids

    Catalogue of Polypents. Enumerations and pictures of these neglected polyforms.
    Catalogue of Cyclic Polypents. Polypents whose cells form a closed loop.
    Figure Eight Polypent Loops. Generalized polypents that cross themselves to form a loop in the shape of a figure eight.
    Unique Polypents With Full Symmetry and Minimum Perimeter. Polypents with full symmetry and the uniquely least possible perimeter for their area.
    Unique Polypents With Star Symmetry and Minimum Perimeter. Polypents with star symmetry and the uniquely least possible perimeter for their area.
    Catalogue of Polyhepts. Some more neglected polyforms.
    Catalogue of Cyclic Polyhepts. Polyhepts whose cells form a closed loop.
    Catalogue of Polyocts. Still more neglected polyforms.
    Catalogue of Polyenns. Highly neglected polyforms.
    Catalogue of Cyclic Polyenns. Polyenns whose cells form a closed loop.
    Catalogue of Polypentagrams. Polyforms formed of pentagrams joined edge to edge.
    Catalogue of Polypennies. Polyforms formed by joining equal disks tangentially.

    Solid Figures

    Catalogue of Unitary Polycubes. Polycubes whose faces are all monominoes.
    Catalogue of Besźel Polycubes. Polycubes whose cells have a preponderance of even coordinates.
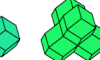    Catalogue of Polyrhons. Polyforms formed by joining rhombic dodecahedrons.
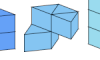    Catalogue of Polyprisms. Polyforms formed by joining equilateral-triangular prisms.
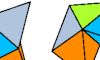    Catalogue of Polytets. Polyforms formed by joining platonic tetrahedra face to face.
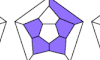    Catalogue of Polypents on the Surface of a Dodecahedron.
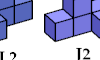    Pentacube Nomenclature. Various systems for naming the 29 pentacubes.
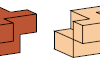    Polycube Symmetries. In how many ways can a polycube be symmetrical?
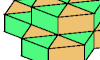    Polyprism Symmetries. In how many ways can a polyprism be symmetrical?
    Catalogue of Polykedges. A polykedge is a polyform formed by joining equal cubes at faces or edges or both.
    Catalogue of Polyominoids. A polyominoid is a polyform formed by joining square cells in the polycube grid.
    Back to Polyform Curiosities.
    Col. George Sicherman [ HOME | MAIL ]